A parallelogon is constructed by two or three pairs of parallel line segments. The vertices and edges on the interior of the hexagon are suppressed.
There are five Bravais lattices in two dimensions, related to the parallelogon tessellations by their five symmetry variations.

In geometry, a parallelogon is a polygon with parallel opposite sides (hence the name) that can tile a plane by translation (rotation is not permitted).[1][2]

Parallelogons have an even number of sides and opposite sides that are equal in length. A less obvious corollary is that parallelogons can only have either four or six sides;[1] Parallelogons have 180-degree rotational symmetry around the center.

A four-sided parallelogon is called a parallelogram.

The faces of a parallelohedron (the three dimensional analogue) are called parallelogons.[2]

Two polygonal types

Quadrilateral and hexagonal parallelogons each have varied geometric symmetric forms. They all have central inversion symmetry, order 2. Every convex parallelogon is a zonogon, but hexagonal parallelogons enable the possibility of nonconvex polygons.

SidesExamplesNameSymmetry
4 ParallelogramZ2, order 2
Rectangle & rhombusDih2, order 4
SquareDih4, order 8
6 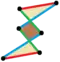Elongated
parallelogram		Z2, order 2
Elongated
rhombus		Dih2, order 4
Regular
hexagon		Dih6, order 12

Geometric variations

A parallelogram can tile the plane as a distorted square tiling while a hexagonal parallelogon can tile the plane as a distorted regular hexagonal tiling.

Parallelogram tilings
1 length 2 lengths
Right Skew Right Skew

Square
p4m (*442)
Rhombus
cmm (2*22)
Rectangle
pmm (*2222)
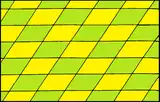Parallelogram
p2 (2222)
Hexagonal parallelogon tilings
1 length 2 lengths 3 lengths
Regular hexagon
p6m (*632)		 Elongated rhombus
cmm (2*22)		 Elongated parallelogram
p2 (2222)

References

  1. 1 2 Aleksandr Danilovich Alexandrov (2005) [1950]. Convex Polyhedra. Translated by N.S. Dairbekov; S.S. Kutateladze; A.B. Sosinsky. Springer. p. 351. ISBN 3-540-23158-7. ISSN 1439-7382.
  2. 1 2 Grünbaum, Branko (2010-12-01). "The Bilinski Dodecahedron and Assorted Parallelohedra, Zonohedra, Monohedra, Isozonohedra, and Otherhedra". The Mathematical Intelligencer. 32 (4): 5–15. doi:10.1007/s00283-010-9138-7. hdl:1773/15593. ISSN 1866-7414. S2CID 120403108. PDF
  • The facts on file: Geometry handbook, Catherine A. Gorini, 2003, ISBN 0-8160-4875-4, p.117
  • Grünbaum, Branko; Shephard, G. C. (1987). Tilings and Patterns. New York: W. H. Freeman. ISBN 0-7167-1193-1. list of 107 isohedral tilings, p.473-481
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.