First Section
This is a Wikiversity article that can be converted on the fly into a presentation.
- Wiki2Reveal persentation of this page
- Every section in this article is a slide in Wiki2Reveal
- All updates in this article will change the Wiki2Reveal persentation
- Slides can have audio comments in the page
Second Section
This is a Wiki2Reveal Demo page for testing embedded audio and video files. In the exported presentation in Reveal you can see a triangle "►". The triangle indicates that currently an audio comment is available for audioplayer below. Click on the triangle to start the audio in the slide mode of Wiki2Reveal.
Third Section
- (Presenter Slides) You can present the slides in you browser as presenter in slides mode by pressing (S)
- (Fullscreen Mode) By pressing (F) in you browser (e.g. Firefox) you see slides in full screen
- (Annotation of Slides) you can annotate slides with a stylus. Press (C) to comment slides. Be aware of the fact, that the annotations are performed in your browser ONLY and all annotations are lost on RELOAD of the page.
Purpose of the Document
This is a Audio slides Wiki2Reveal Test Page that is used to check converting a Wiki page "on the fly" into a reveal presentation.
slide is splitted by audio sample, first Accordion sound
Audio Comments in Section
Audio Comments in the text can be used
- to add additional audio comments to a slide or
- to play audio samples in the reveal presentation
Now a Rock Beat for drums
Splitting Slide with Audio
This slide is splitted by audio sample, first Accordion sound Press play on audio player and hear the accordion sound Again the Rock Beat for drums
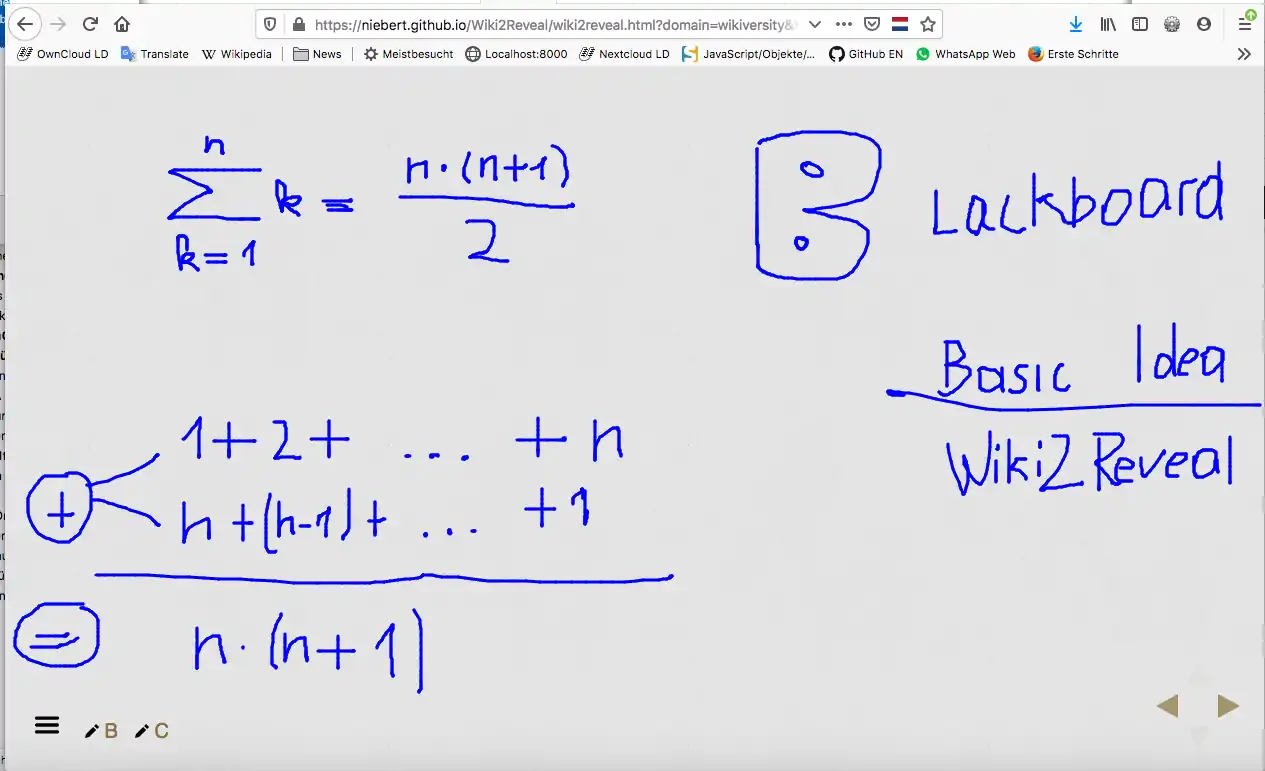
Blackboard for each Page
- You have a blackboard for each page - press (B) to enter the blackboard mode for the slide
- blackboard slide have a grey background and you can write on it for additional comments
- leave blackboard mode - press (B) again
- blackboard comments are performed in your browser ONLY. A comments are not stored for the Wiki2Reveal session and lost on RELOAD.
Inline Math Expression in Slides
The condition that U be simply connected means that U has no "holes" or, in homotopy terms, that the fundamental group of U is trivial; for instance, every open disk qualifies. The condition is crucial; consider
Block Math Expression in Slides
which traces out the unit circle, and then the path integral
is non-zero; the Cauchy integral theorem does not apply here since because the domain of is not a convex set (.
Embedded Video
Video about a gravitational lens Two black holes that rotate.
Annotation/Comments of Slides
Blackboard Screenshot
See also
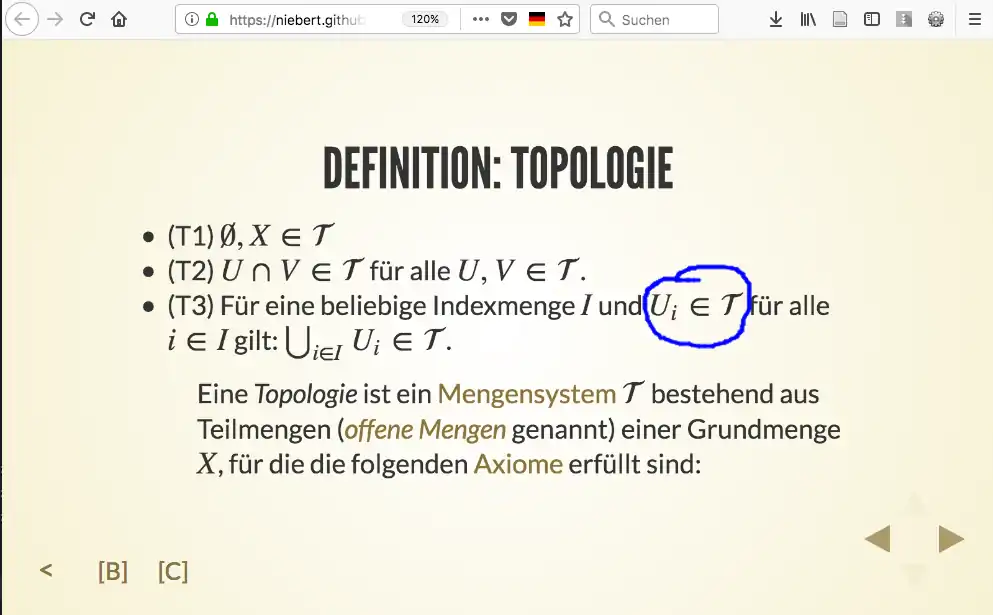
- German Demo Wiki2Reveal presentation - Wikiversity Source: Normen, Metriken, Topologie
- Wiki2Reveal GitHub-Repository - Download GitHub-ZIP for use as AppLSAC-0 - start
docs/index.htmlin your browser.
Wiki2Reveal Information
- Create a Reveal presentation from this article dynamically with Audio slides
- Source Article in Wikiversity
- Create Links for Wiki2Reveal with the Wiki2Reveal Link Creator