Graphing is an important application of derivatives. We take the standard approach, but outline how we can adapt it towards K-6 students.

Motivation

Until now, for linear equations, we graph by plotting points. But for true curves, plotting points can miss directional changes that exists between any 2 points taken. We need a sure way to find ALL direction changes. Our method to find directions y'=0 will cast a wide net, that is, but we are assured trap them all, and catch some inflection points too. That's why we call y'=0 "trapping" all the directional changes because trapping is blunt and imprecise. "Find" is the more precise act of getting just the directional changes.

As shown in the Steps section below, trapping y'=0 finds all the candidate intervals where y' changes between positive & negative signs, or equivalently, where y' crosses the x-axis.

Venn Diagram showing groups of points trapped by y'=0 & y"=0

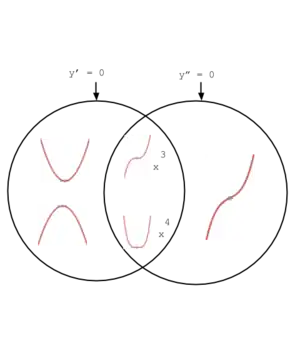
Principles

Tangent line = the line that touches the graph only once, without crossing it.
Tangent slope = slope of tangent line = speed

Positive/negative y' tells the direction that f is moving. For instance, this positive slope shows that the graph must be increasing (regardless of curvature).

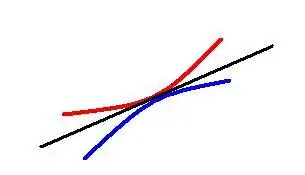
Positive/negative y'' tells the curvature of f (show graphically). For instance, this increasing slope (positive y'') shows that the graph must curve upwards.

Similarly for negative y' and y double prime.

For some students the term "concave up" means a FULL parabola shape of increasing AND decreasing y, rather than a unidirectional half parabola which is also concave up. For lack of a better term, we use the more vivid term "hold water" for concave up and "dump water" for concave down. The idea is that in a drawing a half parabola can also hold water (nevermind the effects of gravity).

Steps

We use the typical Calculus approach, which we summarize here for convenience. To avoid an alphabet soup of letters, we avoid using the additional letter of f(x), and use y(x) instead.

Take the example of -x³+3x²-1. For each graphing problem, students should follow the four steps (a,b,c,d) and produce the 3 figures (x-axis sections, graph, table) below.

These steps are labeled as 0,a,b,c,d in the figure below:
0.  denominator = 0.  if applicable, find the asymtotes in a rational function.
a.  y'=0:  trap    flat    points with y'=0.  plot them.
b.  y'=0:  cut x-axis with flat points           into sections of +/- y' (rising/falling f).  
           on the graph, connect flat points with using rising & falling sections of f.
c.  y"=0:  trap inflection points with y"=0.  plot them.  
d.  y"=0:  CUT x-axis with inflection candidates into SECTIONS of up/down curvatures
           adjust the graph from (b) using proper curvatures.
By "cutting" into regions, we mean that the critical points for y'=0 & y"=0 
divides the domain as follows:
                             d          c              .    d                  
y"                          (+)         0              .   (-)                 
   <------------------------------------+--------------+----------------------->
y'                 (-)                  0      (+)     2            (-)        
step:               b                   a       b      a             b         
                                        .              .                       
                                        .              .                       
                                      3 +           _ -*-                      
                                        |          '   . -                     
                                        |         |    .  |                    
                                        |         |    .   '                   
                                        |        '     .    |                  
                                        |       |      .     '                 
                                        |       |      .      |                
                                        |      *       .                       
                                        |     |        2                       
<--------------------------------'_-----0----*---------+----------------------->
                                   __   |   .          .                       
                                     -. |  _           .                       
                                     -1 **'            .                       
                                        .              .                       
                                        .              .                        
The data table and it's labeled steps are as follows:
       +-----+----+-------+----------+
       |   x | f  | y'    |  y"(x)   |                                          
       +-----+----+-------+----------+
       |     |    |       |          |
    +- |     |    |       |          |                                           
a --|  |  0  | -1 | 0     |          |                                           
    |  |  2  |  3 | 0     |          |                                           
    +- |     |    |       |          |                                           
       |     |    |       |          |                                           
    +- |     |    |       |          |                                           
    |  |  1  |    | +     |   0 <--c |                                           
    |  |     |    |       | +---+    |                                           
b --|  | -1  |    | -     | | + |- d |                                           
    |  |  3  |    | -     | | - |    |                                           
    +- |     |    |       | +---+    |                                           
       |     |    |       |          |                                           
       +---+------+-------+----------+

When graphing, students should produce all 3 figures (graph, table, x-axis cuts)

Exercises

Graphing even a basic polynomial is a complex task for children and can take over an hour, so it's a good idea to break it down to manageable exercises. Here's a sample exercise progression.

1. review solving quadratics
2. a good starter type of exercise is give the students the 3 figures above (table, cuts, graph) and ask them to table the steps a,b,c,d as they occur in the figures.
3. next, given Cuts, Sections, and tables as above, sketch the graph.
4. then, given the formula for f(x), find the regions where f increases/decrease, curves upwards/downwards. eg. find the cuts in the x-axis
5. finally, graph
   • x³ - 3x² + 1
   • 4x³ - 12x²
   • 3x² - 6x
   • 4x / x² + 1.

    see https://www.math.ucdavis.edu/~kouba/CalcOneDIRECTORY/graphingdirectory/Graphing.html

6. once students can graph polynomials, have them graph basic trig functions next sin(x), cos(x). These are trickier and they should review the unit circle. Finding the zero's of sin & cos should be reviewed first.