Radiation
Radiation types
Sun light radiation
Fire radiation
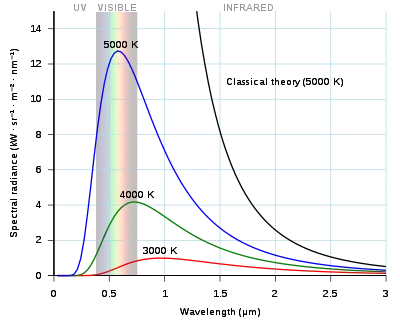
Black Body Radiation
Plank knew that black body absorbs heat better than any other matter . He went on perform an experience on black matter . His experienment shows that
Planck's law of black-body radiation
Planck's law states that
where
- Bν(T) is the spectral radiance (the power per unit solid angle and per unit of area normal to the propagation) density of frequency ν radiation per unit frequency at thermal equilibrium at temperature T.
- h is the Planck constant;
- c is the speed of light in a vacuum;
- k is the Boltzmann constant;
- ν is the frequency of the electromagnetic radiation;
- T is the absolute temperature of the body.
For a black body surface the spectral radiance density (defined per unit of area normal to the propagation) is independent of the angle of emission with respect to the normal. However, this means that, following Lambert's cosine law, is the radiance density per unit area of emitting surface as the surface area involved in generating the radiance is increased by a factor with respect to an area normal to the propagation direction. At oblique angles, the solid angle spans involved do get smaller, resulting in lower aggregate intensities.
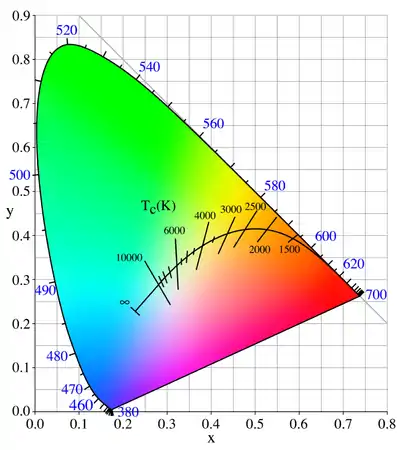
Wien's displacement law
Wien's displacement law shows how the spectrum of black-body radiation at any temperature is related to the spectrum at any other temperature. If we know the shape of the spectrum at one temperature, we can calculate the shape at any other temperature. Spectral intensity can be expressed as a function of wavelength or of frequency.
A consequence of Wien's displacement law is that the wavelength at which the intensity per unit wavelength of the radiation produced by a black body is at a maximum, , is a function only of the temperature:
where the constant b, known as Wien's displacement constant, is equal to 2.8977729(17)×10−3
K m
Planck's law was also stated above as a function of frequency. The intensity maximum for this is given by
- .
Stefan–Boltzmann law
By integrating over the frequency the integrated radiance is
by using with and with being the Stefan–Boltzmann constant. The radiance is then
per unit of emitting surface.
On a side note, at a distance d, the intensity per area of radiating surface is the useful expression
when the receiving surface is perpendicular to the radiation.
By subsequently integrating over the solid angle (where ) the Stefan–Boltzmann law is calculated, stating that the power j* emitted per unit area of the surface of a black body is directly proportional to the fourth power of its absolute temperature:
by using
Alpha radiation
Radiation result from unstable radioactive element like Uranium (Ur) decays to becomes more stable element Thorium (Th) and release alpha radiation , alpha photon of quantum's energy
- Ur --> Th + alpha radiation
Beta radiation
Radiation result from unstable isotope element like Carbon (C) decays to becomes more stable element Nitrogen (N) and release beta radiation , beta photon of quantum's energy
- C --> N + beta radiation
Gamma radiation
Electromagnetic radiation
All radiations can be best understand as electromagnetic oscillation wave of two fields Electric field and Magnetic field perpendicular to each other that has wave function
Wave travels at speed equals to wave's angular speed that has value of speed of visible light
Electromagnetic radiation and matter
Radiation interact with matter to create Heat transfer of three phases Heat conduction, Heat convection and Heat radiation
Heat conduction
Matter absorbs photon's energy and release heat into the surrounding
Heat convection
Matter absorbs photon's energy to the mazximum at Threshold frequency fo and release visible light into the surrounding
Heat radiation
Matter's atom releases its electron into the surrounding at frequency greater than threshold frequency fo