When calculation the sum of all voltage drops as ${\displaystyle \sum _{j}\Delta V_{j}=0}$, attention must be paid to the direction assigned to the stated current through a resistor or voltage source.

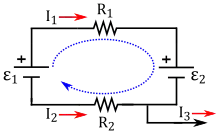
The figure can be used to create two equations involving the currents and voltages: Starting at ${\displaystyle \varepsilon _{1}}$ and going through ${\displaystyle R_{1}}$ indicated by the dotted blue path, we have, by conservation of potential energy:

${\displaystyle \varepsilon _{1}-I_{1}R_{1}-\varepsilon _{2}+I_{2}R_{2}=0.}$

Note the minus sign that results at ${\displaystyle R_{1}}$ when the path follows the direction of the stated current. Also note how the sign associated with ${\displaystyle \varepsilon _{1}}$ and ${\displaystyle \varepsilon _{2}}$ depends on the orientation of the voltage sources with respect to the path.

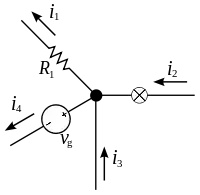
By conservation of charge, at the juncture (node) where the three currents meet near the bottom right corner, we have:

${\displaystyle I_{1}+I_{2}=I_{3}}$.

It should be emphasized that if both stated voltages ${\displaystyle (\varepsilon _{1},\varepsilon _{2})}$ are positive, we do not know the direction of the actual current at ${\displaystyle R_{1}}$. If further calculations show that ${\displaystyle I_{1}<0}$, we conclude that the actual current is travelling in the direction opposite to the red arrow shown in the figure.

Kirchhoff's current law

The sum of all currents entering a node is equal to the sum of all currents leaving the node.

Kirchhoff's voltage law

The directed sum of the electrical potential differences around a loop must be zero.