Conic sections are curves created by the intersection of a plane and a cone. There are six types of conic section: the circle, ellipse, hyperbola, parabola, a pair of intersecting straight lines and a single point.

All conics (as they are known) have at least two foci, although the two may coincide or one may be at infinity. They may also be defined as the locus of a point moving between a point and a line, a directrix, such that the ratio between the distances is constant. This ratio is known as "e", or eccentricity.

Ellipses

Animation showing distance from the foci of an ellipse to several different points on the ellipse.

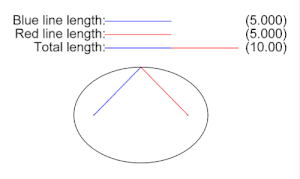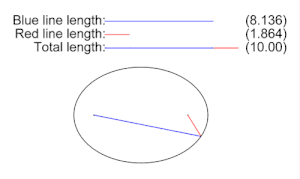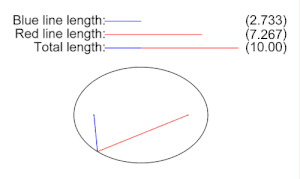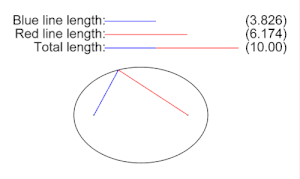
An ellipse is a locus where the sum of the distances to two foci is kept constant. This sum is also equivalent to the major axis of the ellipse - the major axis being longer of the two lines of symmetry of the ellipse, running through both foci. The eccentricity of an ellipse is less than one.

In Cartesian coordinates, if an ellipse is centered at (h,k), the equation for the ellipse is

${\displaystyle {\frac {(x-h)^{2}}{a^{2}}}+{\frac {(y-k)^{2}}{b^{2}}}=1}$    (equation 1)

The lengths of the major and minor axes (also known as the conjugate and transverse) are "a" and "b" respectively.

Exercise 1. Derive equation 1.    (hint)

A circle circumscribed about the ellipse, touching at the two end points of the major axis, is known as the auxiliary circle. The latus rectum of an ellipse passes through the foci and is perpendicular to the major axis.

From a point P(${\displaystyle x_{1}}$, ${\displaystyle y_{1}}$) tangents will have the equation:

${\displaystyle {\frac {xx_{1}}{a^{2}}}+{\frac {yy_{1}}{b^{2}}}=1}$

And normals:

${\displaystyle {\frac {xa^{2}}{x_{1}}}-{\frac {yb^{2}}{y_{1}}}=a^{2}-b^{2}}$

Likewise for the parametric coordinates of P, (a ${\displaystyle \cos \alpha }$, b ${\displaystyle \sin \alpha }$),

${\displaystyle {\frac {x\cos \alpha }{a}}+{\frac {y\sin \alpha }{b}}=1}$

Properties of Ellipses

S and S' are typically regarded as the two foci of the ellipse. Where ${\displaystyle a>b}$, these become (ae, 0) and (-ae, 0) respectively. Where ${\displaystyle a<b}$ these become (0, be) and (0, -be) respectively.

A point P on the ellipse will move about these two foci ut ${\displaystyle |PS+PS'|=2a}$

Where a > b, which is to say the Ellipse will have a major-axes parallel to the x-axis:

${\displaystyle b^{2}=a^{2}(1-e^{2})}$

The directrix will be: ${\displaystyle x=\pm {\frac {a}{e}}}$

Circles

A circle is a special type of the ellipse where the foci are the same point.

Hence, the equation becomes:

${\displaystyle x^{2}+y^{2}=r^{2}}$

Where 'r' represents the radius. And the circle is centered at the origin (0,0)

Hyperbolas

A special case where the eccentricity of the conic shape is greater than one.

Centered at the origin, Hyperbolas have the general equation:

${\displaystyle {\frac {x^{2}}{a^{2}}}-{\frac {y^{2}}{b^{2}}}=1}$

A point P on will move about the two foci ut ${\displaystyle |PS-PS'|=2a}$

The equations for the tangent and normal to the hyperbola closely resemble that of the ellipse.

From a point P(${\displaystyle x_{1}}$, ${\displaystyle y_{1}}$) tangents will have the equation:

${\displaystyle {\frac {xx_{1}}{a^{2}}}-{\frac {yy_{1}}{b^{2}}}=1}$

And normals:

${\displaystyle {\frac {xa^{2}}{x_{1}}}+{\frac {yb^{2}}{y_{1}}}=a^{2}+b^{2}}$

The directrixes (singular directrix) and foci of hyperbolas are the same as those of ellipses, namely directrixes of ${\displaystyle x=\pm {\frac {a}{e}}}$ and foci of ${\displaystyle (\pm ae,0)}$

The asymptotes of a hyperbola lie at ${\displaystyle y=\pm {\frac {b}{a}}x}$

Rectangular Hyperbolas

Rectangular Hyperbolas are special cases of hyperbolas where the asymptotes are perpendicular. These have the general equation:

${\displaystyle xy=c}$

Other resources

• Should the contents of this Wikiversity page be merged into the related Wikibooks modules such as b:Conic Sections/Ellipse?