A polynomial function is a function compromising of more than one non-negative integer powers of x. An example of this would be:

• 3x² + 4x - 5
• 8x⁴ + 12x³ - 2x² + 5x - 5
• 6x⁷ - 5

A degree is determined by whichever power is the highest. Example: The first polynomial, 3x² + 4x - 5, has ${\displaystyle 2}$ as the highest degree since it is the highest power in the polynomial. The leading coefficient is the coefficient with the highest degree (not value). Example: The second polynomial, 8x⁴ + 12x³ - 2x² + 5x - 5, has "8" as its leading coefficient since it is the number that is associated with the highest degree, being "4".

A quadratic is a polynomial with the highest degree being ${\displaystyle 2}$. A cubic polynomial, or simply "cubic", is a polynomial with the highest degree being ${\displaystyle 3}$.

Graphs

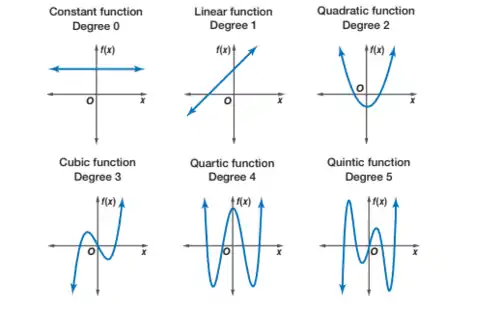
Watch closely how the degrees are related to the zeroes of the function (the intersection of the x-axis by the graph). They seem to correlate each other (be the exact same as each other).

Observe the end behavior. Which direction does x or f(x) lead to? Positive or negative infinity (take note that the functions go infinity and never stop at a certain point)? This is represented by x→+∞ and x→−∞ (x-approaching positive infinity and x-approaching negative identity, respectively). The degree and leading coefficient of a polynomial function determine the end behavior of the polynomial function graph. See Varsity Tutors - End Behavior of a Function (examples) for more examples of end behavior of functions.

• If the x-axis was intersected twice or thrice, it has two/three real zeroes.
• If the x-axis was not intersected at all, its roots are imaginary.
• If the function was tangent to the x-axis, this means it is a double root, which represents two zeroes at the same number (x-axis point).

A function is even if both sides of the function go the same direction, while a function is odd if both sides of the function go in opposite directions.

You determine the number of degrees and the total number of solutions for a function by counting the turns of the graph and adding one to it.