Page:Calculus Made Easy.pdf/235

This page has been proofread, but needs to be validated.

FINDING AREAS BY INTEGRATING

215

(3) Here’s another case–that of the die-away curve (p. 156). Find the area between $x=0$ and $x=a$ , of the curve (Fig. 57) whose equation is

${\begin{aligned}y&=b\epsilon ^{-x}.\\{\text{Area}}&=b\int _{x=0}^{x=a}\epsilon ^{-x}\times dx.\\\end{aligned}}$

The integration (p. 201) gives

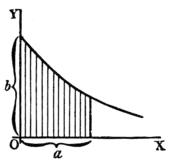${\begin{aligned}&=b\left[-\epsilon ^{-x}\right]_{0}^{a}\\&=b{\bigl [}-\epsilon ^{-a}-(-\epsilon ^{-0}){\bigr ]}\\&=b(1-\epsilon ^{-a}).\end{aligned}}$


Fig. 57.	Fig. 58.

(4) Another example is afforded by the adiabatic curve of a perfect gas, the equation to which is $pv^{n}=c$ , where $p$ stands for pressure, $v$ for volume, and $n$ is of the value $1.42$ (Fig. 58).

Find the area under the curve (which is proportional

This article is issued from Wikisource. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.