ROOT. The classification of the chords which form the structural material of modern harmonic music is attained by referring them to what are called their roots; and it is mainly by their use that these harmonic elements are brought within the domain of intelligible order.
As long as the purely polyphonic system was in full force, the chordal combinations were merely classified according to recognized degrees of consonance and dissonance, without any clear idea of relationship: but as that system merged by degrees into the harmonic system, it was found that fresh principles of classification were indispensable; and that many combinations which at first might appear to have quite a distinct character must somehow be recognised as having a common centre. This centre was found in an ultimate bass note, namely, the bass note of the complete chord in what would be considered its natural or first position; and this was called the Root, and served as the common indicator of all the various portions of the complete chord which could be detached, and their test of closest possible relationship. Further, these roots were themselves classified according to their status in any given key; and by this means a group of chords which were related to one another most closely by having the same root, might be shown to be related severally and collectively to the group which belonged to another root; and the degree of relationship could be easily and clearly ascertained according to the known nearness or remoteness of the roots in question. By this means the whole harmonic basis of a piece of music can be tested; and it must be further noted that it is only by such means that the structural principles of that kind of music which has been called 'absolute' because of its dissociation from words, is rendered abstractedly intelligible.
The principle upon which modern Instrumental Music has been developed is that a succession of distinct tunes or recognizable sections of melody or figures can be associated by the orderly distribution of harmonies and keys in such a manner that the mind can realise the concatenation as a complete and distinct work of art. It is obvious that fine melodic material is a vital point; but it is not so obvious that where the dimensions of the work are such that a continuous flow of melody of a uniform character is impossible, the orderly arrangement of the materials in successions of keys and harmonies is no less vital. The harmonic structure requires to be clearly ascertainable in works of art which are felt to be masterpieces of form, and to be perfectly understood and felt by those who attempt to follow such models: hence, in discussing the structure of works of this kind, the frequent use of such terms as Tonic, or Dominant or Subdominant harmony, which is only a short way of describing harmony of which these respective notes are the roots.
The simplest and most stable of complete combinations in music are the chords consisting of a bass note with its third and perfect fifth; and of these the bass note is considered the root. In most cases such a root is held to be the fundamental sound of the series of harmonics which an essential chord may be taken to represent. For instance, the chord of the major third and perfect fifth on any note is supposed to represent the ground tone or generator with two of its most distinct and characteristic lower harmonics; and whatever be the positions of the individual notes in respect of one another, they are still referred to this ground-tone as a root. Thus the chord GBD (a) would be taken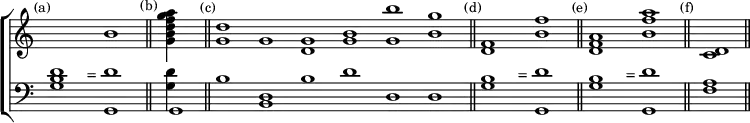
to be the representative of the ground-tone G with its second and fourth harmonics (b); and every transposition or 'inversion' of the same notes, such as BDG, or DGB in close or open order (as in c), or even lesser portions to which the implication of a context would afford a clue, would be referred alike to this same root. If F be added (d) to the above chord it may be taken to represent the sixth harmonic (b), and similar 'inversions' of the component portions of the chord will similarly be referred to the note G. If A be added further above the F of the preceding chord, producing G B D F A (as in e), that is commonly taken as a yet more complete representation of the group of harmonics generated by the sounding of G, of which it is the eighth; and, as before, all the different portions which could be intelligibly isolated, and all the transpositions of its component notes, would be still referable to the one root G. If A♭ had been taken instead of A♮, the same general explanation would hold good, though the special question might remain open whether it was a representative of the 16th harmonic, which is four octaves from the fundamental sound, or an artificial softening of the clear and strong major ninth, A♮. Some theorists carry the same principles yet further, and include the C above A, and even the E and E♭ above that in the group which represents the harmonic series of G, calling them respectively the eleventh and major and minor thirteenths of that note.
The discords contained in the above series are frequently styled fundamental, from this supposed representation of the group of harmonics generated by their fundamental or root note; they are characterised among discords by the peculiar freedom of the notes of which they are composed, on both sides. It will be observed that they are all members of the Diatonic series of the key of C, major or minor; and as G, their root note, is the Dominant of that key, they represent the scope of what is called the Dominant harmony of C, which of course has its counterpart in every other key. No other note than the Dominant serves to this extent as the root of chords of this class which are Diatonic. The Tonic, for instance, can only supply the third and fifth, and even the minor seventh is a chromatic note. Nevertheless this chromatic chord and the ninths which are built upon it are commonly used as if they belonged to the key of C; and the same remark applies to the similar discords founded on the Supertonic root (as D in the key of C); and these are most readily intelligible through their close connection as Dominant harmony to the Dominant of C.
The roots of the various combinations which are arrived at by modifying the intervals of such distinct and essential harmonies as the above, are of course the same as those of the unmodified harmonies. Thus the roots of suspensions are the same as those of the harmonies upon which they are said to resolve, because they are modifications of that which follows in its complete state, and not of that which precedes; and the same applies to the combinations produced by adventitious notes, such as appoggiaturas and the like.
The combinations which arise from the simultaneous occurrence of ordinary passing notes must find their root in the chord which precedes, as that has possession of the field till new harmony presents itself.
From these considerations it will be obvious that a very considerable variety of apparently different combinations are referable to a single root. In fact a great portion of music is built upon very few roots; many examples of good popular music especially do not exceed the limits of Tonic and Dominant harmony with an occasional move as far as the Sub-dominant, and next to no modulation. Even in works which belong to the domain sometimes distinguished as high art a great deal is often done within very narrow limits. For instance, the whole of the first section of a violin and pianoforte sonata of Mozart's in A is based on six successive alternations of Tonic and Dominant harmony, and modulation to the new key for the second section is effected merely by the Dominant and Tonic harmony of that key.
Notwithstanding the importance which attaches to a clear understanding of the classification of chords according to their roots, there are some combinations upon whose derivation doctors disagree; and it must be confessed that the theory of music is yet far from that complete and settled stage which would admit any hope of a decisive verdict in the matter at present. In such circumstances variety of opinion is not only inevitable but desirable; and though the multitude of counsellors is a little bewildering there are consolations; for it happens fortunately that these differences of opinion are not vital. Such chords, for instance, as augmented sixths have so marked and immediate a connection with the most prominent harmonies in the key, that the ascertainment of their roots becomes of secondary importance; and even with the chord which stands as in the key of C for instance (f), it is not so indispensable to decide whether G or F or D is the root, or whether indeed it is even a double-rooted chord, because, among other reasons, the very attention which has been called to it and the very characteristics which have made it difficult to classify have given it a prominence and a unique individuality which relieves it of the need of being assigned to any category; and even when it is an important factor in the harmonic structure, the process of analysis need not be rendered doubtful because its actual position in the key is so thoroughly realised. Other disputed points there are having reference to roots, which are even of less importance. For instance, whether what is called an augmented fifth is really an augmented fifth or a minor thirteenth; or whether the augmented octave which Mozart uses with such marked emphasis in the 3rd bar of the Allegro in the overture to Don Giovanni is properly a minor ninth, as some maintain—since happily the roots would be the same in both cases.[ C. H. H. P. ]