CHANGE. I. The word used as the short for change of key or Modulation, under which latter head a fuller account is given. Changes are commonly spoken of as of three kinds, representing three degrees of abruptness.
1. The Diatonic, which passes from one key to another, nearly related to it, by means of notes common to both, as—
![{ \override Score.TimeSignature #'stencil = ##f \time 2/4 \key d \major \partial 8 << \relative f'' << { fis8 | b,32 cis d8. cis32 b ais8. | b8[ a16 g fis8] fis' | g( fis) fis( e) | a( g16 fis) e8 d-. | <e b g>4 cis | <d fis,> } \\ { fis,8 | d4 g8 fis | d[ e d] b' | <d b>4 <cis a> | <d a> r8 <b fis> | s4 e, } >>
\new Staff { \clef bass \key d \major \relative b << { b8 b4 cis8 ais } \\ { <d, b>8 g fis <g e> fis } >> <fis b,> <e cis> <fis d> <b b,> | <e e,>4 <a a,> | <fis fis,> r8 <b b,> | <g g,>4 <a a,> | <d d,> } >> }](../../I/5106d8f6ec5bbb38eae329f89c7ac335.png.webp)
from Bach's Cantata, 'Freue dich, erlöste Schaar.'
2. The Chromatic, when accidentals appear which are not common to both keys, as—
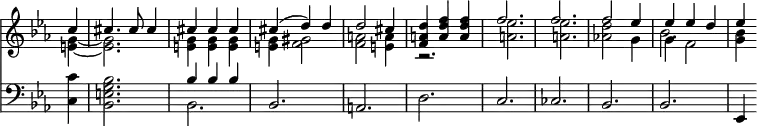
from Mozart's Requiem.
3. The Enharmonic, where advantage is taken of the fact that the same notes can be called by different names, which lead different ways, and consequently into unexpected keys. For instance, the dominant 7th can be translated into the chord of the augmented 6th, and by that means lead into very remote keys, and by the universal transformable power of the inversions of the minor 9th, we can pass from any one key to almost any other; e.g. in Beethoven's 'Leonore' Overture the transition from E major to F is thus managed—
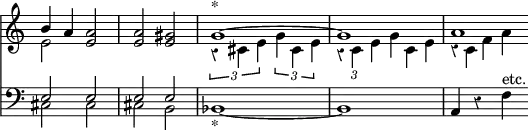
the chord * begin resolved as if it had been written B♭, D♭, G, and being approached as if it should be written A♯, C♯, G. Thus there is a double equivoque. The chord as it is approached seems to be an inversion of the minor 9th of the supertonic of E; it is then written as an inversion of the chord of the minor 9th of the dominant in the key of D, and resolved as an inversion of the minor 9th of the dominant of F. A more obvious instance to the uninitiated is the following—
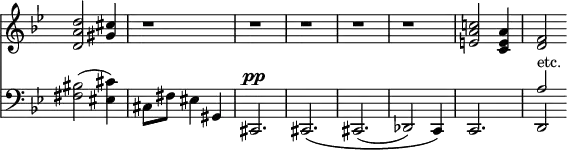
[ C. H. H. P. ]
II. Change is the term applied to any order in which bells are struck other than the usual order in which rings of bells are arranged, viz. the diatonic scale—struck from the highest to the lowest bell; and Change Ringing[1] is the continual production of such changes—without any repetition—from the time the bells leave the position of rounds (1 2 3 4 5 6) to the time they return to that position again. It is an interesting, and, to many, an engrossing art, and has been in practice in this country, it is supposed, for the last 250 years; during which time many persons of rank and education have practised it as an amusement, among the earliest of whom may be mentioned Lord Brereton, and Sir Cliff Clifton in about 1630. Change ringing, as has been said, is the constant production of changes without repetition from the time that the bells leave the position of rounds to the time that they return to that position again. It is a rule that every bell which can change its position should do so in order of striking at each successive blow, thus:—
| 1 2 3 4 5 |
| 2 1 4 3 5 |
| 2 4 1 5 3 |
It is the change ringers' and the composer's object to obtain with as musical a combination as may be, the whole of the changes to be produced on any given number of bells. It will be seen by examining the following figures that with this simple rule—that every bell which can must change places—only 10 changes can be produced on five bells
1 2 3 4 5
2 1 4 3 5
2 4 1 5 3
4 2 5 1 3
4 5 2 3 1
5 4 3 2 1
5 3 4 1 2
3 5 1 4 2
3 1 5 2 4
1 3 2 5 4
1 2 3 4 5
It will also be observed that the bells work in regular order from being first bell to being last, striking two blows as first and two as last: this is called by ringers 'hunting up and down'—all the work from being first bell being called hunting 'up,' till she becomes the last striking bell, and the reverse being termed going 'down.' A bell can never be made to skip a place, she must always be rung in the next place to that in which she last struck. This being the rule, therefore, that bells must thus change places, and it having been shown that by simply doing so only 10 changes of the 120 on five bells (see Table) can be produced, it becomes necessary to alter the rule in the case of some of the bells, by making fresh ones; and these rules, being more or less intricate, comprise the methods by which peals or touches are produced. For the purposes of this work it will be enough to glance at one or two of those in most general use.
The Grandsire method is supposed to be the original one, and shall therefore be first noticed. Taking the rule above given as to plain 'hunting,' and which has been shown to produce ten changes only on five bells, it is by this method thus altered:—The bell that leads next before the treble only goes up into 3rd's place and then goes back to lead again; the bells in fourths and fifths places are by this thrown out of their work, as will be seen by the following diagram at the asterisk, and are said to dodge:—
1 2 3 4 5
2 1 3 5 4
2 3 1 4 5
3 2 4 1 5
3 4 2 5 1
4 3 5 2 1
4 5 3 1 2
5 4 1 3 2†
5 1 4 2 3
1 5 2 4 3
1 2 5 3 4*
2 1 5 4 3
2 5 1 3 4
5 2 3 1 5
5 3 2 4 1
By following this rule again only 30 changes of the 120 can be produced, and now the services of the conductor have to be called in, who uses the terms 'Bob' or 'Single' to denote the changes in work shown in the following diagrams, taking up the work from the † in the foregoing one. We will in the first show the working of a Bob, in the second that of a Single,—these changes of course always taking place when the treble is leading:—
'Bob' 5 4 1 3 2
5 1 4 2 3
1 5 4 3 2
1 4 5 2 3
4 1 5 3 2
4 5 1 2 3
'Single' 5 4 1 3 2
5 1 4 2 3
1 5 4 3 2
1 5 4 2 3
5 1 4 3 2
5 4 1 2 3
It will be observed that all the bells, except the treble, are thrown out of their plain hunting work; the 4th and 5th remain below 3rd's place, and the 2nd and 3rd keep changing places: in change-ringing terms the 4th and 5th are said to 'make places,' and the 2nd and 3rd are said to make a 'double dodge.' It is by calling these bobs and singles at intervals previously settled on that the conductor is able to produce the whole 120 changes.
This method is much and generally practised on all numbers of bells from 5 to 12, its working being exactly the same on all, with the only difference that when the courses of the bells are altered by the rule, there are more bells to dodge, and the arrangements of bobs and singles become more complicated. It is, however, considered better suited to an uneven number of bells with a tenor covering,—such as would be ten bells when only the first nine were changing.
The Stedman method is another and favourite method among change-ringers. It derives its name from a Mr. Fabian Stedman by whom it was invented about the year 1640. It is on an entirely different principle to the Grandsire method, the foundation of it being that the three first bells go through the six changes of which they are capable (see Table of Changes) while the bells behind 'dodge'; at the end of each six changes one of these bells going up to take part in the dodging, and another coming down to take its place in the changes. It is an intricate method, and our space will not allow of a fuller explanation; it is carefully explained in Troyte's 'Change Ringing,' to which we have already referred.
Treble Bob. There are many variations of this which is usually performed on an even number of bells. It derives its name from the fact that, instead of the plain hunting course, the bells, and more especially the 'Treble,' have a dodging course. This will be seen by the following diagram, and for further explanation we must again refer to Troyte's 'Change Ringing.'
1 2 3 4 5 6
2 1 3 4 6 5
1 2 4 3 5 6
2 1 4 3 6 5
2 4 1 6 3 5
4 2 6 1 5 3
4 2 1 6 3 5
2 4 6 1 5 3
2 6 4 5 1 3
6 2 5 4 3 1
6 2 4 5 1 3
2 6 5 4 3 1
5 2 3 6 1 4
5 2 6 3 4 1
2 5 3 6 1 4
2 3 5 1 6 4
3 2 1 5 4 6
3 2 5 1 6 4
2 3 1 5 4 6
2 1 3 4 5 6
1 2 3 4 6 5
2 1 4 3 5 6
1 2 4 3 6 5
1 4 2 6 3 5
1 4 6 2 3 5
4 1 6 2 5 3
4 6 1 5 2 3
The foregoing remarks we trust will explain the general meaning of the term 'Change Ringing' as used technically. The following Table shows the number of changes to be derived from any given number of bells up to 12 (the largest number ever rung in peal), the names given to such changes, and the time generally allowed for ringing them:—
| No. of Bells. | Name. | No. of Changes. | Years. | Days. | Hours. | Minutes |
|---|---|---|---|---|---|---|
| 3 | . . . . . . . | 6 | . . | . . | . . | . . |
| 4 | Singles . . . | 24 | . . | . . | . . | 1 |
| 5 | Doubles . . . | 120 | . . | . . | . . | 5 |
| 6 | Minor . . . | 720 | . . | . . | . . | 30 |
| 7 | Triples . . . | 5,040 | . . | . . | 3 | . . |
| 8 | Major . . . | 40,320 | . . | 1 | 4 | . . |
| 9 | Caters (quaters) | 362,880 | . . | 10 | 12 | . . |
| 10 | Royal . . . | 3,628,800 | . . | 105 | . . | . . |
| 11 | Cinques . . . | 39,916,800 | 3 | 60 | . . | . . |
| 12 | Maximus . . . | 479,001,600 | 37 | 355 | . . | . . |
[ C. A. W. T. ]
- ↑ This work being a Dictionary of Music, a long description of the art would be out of place, and we must therefore refer the reader to the elementary book entitled 'Change Ringing' by Charles A. W. Troyte. Esq., of Honisham, Devon (Masters, New Bond Street), and for the more advanced stages to the book of the same name by Mr. William Banister (Pollard, Exeter).