NAVIGATION (from Lat. navis, ship, and agere, to move), the science or art of conducting a ship across the seas. The term is also popularly used by analogy of boats on rivers, &c., and of flying-machines or similar methods of locomotion. Navigation, as an art applied properly to ships, is technically used in the restricted sense dealt with below, and has therefore to be distinguished from “seamanship” (q.v.), or the general methods of rigging a ship (see Rigging), or the management of sails, rudder, &c.
History.
The early history of the rise and progress of the art of navigation is very obscure, and it is more easy to trace the gradual advance of geographical knowledge by its means than the growth of the practical methods by which this advance was attained. Among Western nations before the introduction of the mariner's -compass the only practical means of navigating ships was to keep in sight of land, or occasionally, for short distances, to direct the ship's course by referring it to the sun or stars; this very rough mode of procedure failed in cloudy weather, and even in short voyages in the Mediterranean in such circumstances the navigator generally became hopelessly bewildered as to his position.
Over the China Sea and Indian Ocean the steadiness in direction of the monsoons was very soon observed, and by running directly before the wind vessels in those localities were able to traverse long distances out of sight of land in opposite directions at different seasons of the year, aided in some cases by a rough compass (q.v.). But it is surprising when we read of the progress made among the ancients in fixing positions on shore by practical astronomy that so many years should have passed without its application to solving exactly the same problems at sea, but this is probably to be explained by the difficulty of devising instruments for use on the unsteady platform of a ship, coupled with the lack of scientific education among those who would have to use them.
The association of commercial activity and nautical progress shown by the Portuguese in the early part of the 15th century marked an epoch of distinct progress in the methods of practical navigation, and initiated that steady improvement which in the zoth century has raised the art of navigation almost to the position of an exact science. Up to the time of the Portuguese exploring expeditions, sent out by Prince Henry, generally known as the “Navigator,” which led to the discovery of the Azores in 1419, the rediscovery of the Cape Verde Islands in 1447 and of Sierra Leone in 1460, navigation had been conducted in the most rude, uncertain and dangerous manner it is possible to conceive. Many years had passed without the least improvement being introduced, except the application of the magnetic needle about the beginning of the 14th century (see Compass and Magnetism). Prince Henry did all in his power to bring together and systematize the knowledge then obtainable upon nautical affairs, and also established an observatory at Sagres (near Cape St Vincent) in order to obtain more accurate tables of the declination of the sun. John II., who ascended the throne of Portugal in 1481, followed up the good work. He employed Roderick and Joseph, his physicians, with Martin de Bohemia, from Fayal, to act as a committee on navigation. They calculated tables of the sun's declination, and improved the astrolabe, recommending it as more convenient than the cross-staff. The Ordenanzas of the Spanish council of the Indies record the course of instruction prescribed at this time for pilots; it included the De Sphaera Mundi of Sacrobosco, the spherical triangles of Regiomontanus, the Almagest of Ptolemy, the use of the astrolabe and its mechanism, the adjustments of instruments, cartography and the methods of observing the movements of heavenly bodies.
The then backward state of navigation is best understood from a sketch of the few rude appliances which the mariner had, and even these were only intended for the purpose of ascertaining the latitude. The mystery of finding the longitude proved unfathomable for many ears after the time of the Armada, and the very inaccurate knowledge existing of the positions of the heavenly bodies themselves fully justified the quaintly expressed advice given in a nautical work of repute at the time, where the writer observes, “Now there be some that are very inquisitive to have a way to get the longitude, but that is too tedious for seamen, since it requireth the deep knowledge of astronomy, wherefore I would not have any man think that the longitude is to be found at sea by any instrument; so let no seamen trouble themselves with any such rule, but (according to their accustomed manner) let them keep a perfect account and reckoning of the way of their ship.” Such record of the “way of the ship” appears to have been then and for many years later recorded in chalk on a wooden board (log board), which folded like a book, and from which each day a position for the ship was deduced, or from which the more careful made abstracts into what was termed the “journal.”
A compass, a cross-staff or astrolabe, a fairly good table of the sun's declination, a correction for the altitude of the pole star, and occasionally a very incorrect chart formed all the appliances of a navigator in the time of Columbus, For a knowledge of the speed of the ship one of the earliest methods of actual measurement in use was by what was known as the “Dutchman's log,” which consisted in throwing into the water, from the bows of tire ship, something which would float, and noting the interval between its apparently drifting past two observers standing on the deck at a known distance apart. No other method is mentioned until 1577, when a line was attached to a small log of wood, which was thrown overboard, and the length measured which was carried over in a certain interval of time; this interval of time was, we read, generally obtained by the repetition of certain sentences, which were repeated twice if the ship were only moving slowly. It is unfortunate that the words of this ancient shibboleth are unknown. This is mentioned by Purchas as being in occasional use in 1607, but the more usual method (as we incidentally see in the voyages of Columbus) was to estimate or guess the rate of progress. It was customary by one or other of these methods to determine the speed of a ship every two hours, “royal” ships and those with very careful captains doing so every hour. When a vessel had been on various courses during the two hours, a record of the duration on each was usually kept by the helmsman on a traverse board, which consisted of a board having 32 radial lines drawn on it representing the points of the compass, with holes at various distances from the centre, into which pegs were inserted, the mean or average course being that entered on the log board.
Some idea of the speed of ordinary ships in those days may be gathered from an observation in 1551 of a “certain shipp which, without ever striking sail, arrived at Naples from Drepana, in Sicily, in 37 hours” (a distance of 200 m.); the writer accounting for “such swift motion, which to the common sort of man seemeth incredible,” by the fact of the occurrence of “violent Hoods and outrageous winds.” In 1578 we find in Bourne's Inventions and Devices a description of a proposed patent log for recording a vessel's speed, the idea (as far as we can gather from its vague description) being to register the revolutions of a wheel enclosed in a case towed astern of a ship (see Log).
Whether the property of the lodestone was independently discovered in Europe or introduced from the East, it does not appear to have been generally utilized in Europe earlier than about A.D. 1400 (see Compass). In Europe the card or “flie” appears to have been attached to the magnet from the first, and the whole suspended as now in gimbal-rings within the “bittacle,” or, as we now spell the word, “binnacle.” The direction of a ship's head by compass was termed how she “capes.” From the accounts extant of the stores supplied to ships in 1588, they appear to have usually had two compasses, costing 3s. 4d. each, which were kept in charge by the boatswain. The filet that the north point of a compass does not, in most places, point to the true pole but eastward or westward of it, by an amount which is termed by sailors “variation,” appears to have been noticed at an early date; but that the amount of variation varied in different localities appears to have been first observed by either Columbus or Cabot about 1490, and we find it used to be the practice to ascertain this error when at sea either from a bearing of the pole star, or by taking a mean of the compass bearings of the sun at both rising and setting, the deviation of the compass in the ships of those days being too small a quantity to be generally noticed, though there is a very suggestive remark on the effect of moving the position of any iron placed near a compass, by a Captain Sturmy of Bristol in 1679. In order, partially to obviate the error of the compass (variation), the magnets, which usually consisted of two steel wires joined at both ends and opened out in the middle, were not placed under the north and south line of the compass card, but with the ends about a point eastward of north and westward of south, the variation in London when first observed in 1580 being about 11° E.; the change of the variation year by year at the same base was first noted by Gellibrand in 1635.
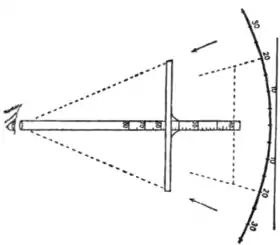
The “cross-staff” appears to have been used by astronomers at a very early period, and, subsequently by seamen for measuring altitudes at sea. It was one of the few instruments possessed by Columbus and Vasco da Gama. The old cross-staff, called by the Spaniards “ballestilla,” consisted of two light battens. The part we may call the staff was about 112 in. square and 36 in. long. The cross was made to fit closely and to slide upon the staff at right angles; its length was a little over 26 in., so as to allow the “pinules” or sights to be placed exactly 26 in. apart. A sight was also fixed on the end of the staff for the eye to look through so as to see both those on the cross and the objects whose distance apart was to be measured. It was made by describing the angles on a table, and laying the staff upon it (fig. 1). The scale of degrees was marked on the upper face. Afterwards shorter crosses were introduced, so that smaller angles could be taken by the same instrument. These angles were marked on the sides of the staff.
To observe with this instrument a meridian altitude of the sun the bearing was taken by compass, to ascertain when it was near the meridian; then the end of the long staff was placed close to the observer’s eye, and the transversary, or cross, moved until one end exactly touched the horizon, and the other the sun’s centre. This was continued until the sun dipped, when the meridian altitude was obtained.
 |
| Fig. 1. |
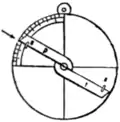
Another primitive instrument in common use at the beginning of the 16th century was the astrolabe (q.v.), which was more convenient than the cross-staff for taking altitudes. Fig. 2 represents an astrolabe as described by Martin Cortes. It was made of copper or tin, about 14 in. in thickness and 6 or 7 in. in diameter, and was circular except at one place, where a projection was provided for a hole by which it was suspended. Weight was considered desirable in order to keep it steady when in use. The face of the metal having been well polished, a plumb line from the point of suspension marked the vertical line, from which were derived the horizontal line and centre. The upper left quadrant was divided into degrees. The second part was a pointer pt of the same metal and thickness as the circular plate, about 112 in. wide, and in length equal to the diameter of the circle. The centre was bored, and a line was drawn across it the full length, which was called the line of confidence. On the ends of that line were fixed plates, s, s, having each a small hole, both exactly over the line of confidence, as sights for the sun or stars. The pointer moved upon a centre the size of a goose quill. When the instrument was suspended the pointer was directed by hand to the object, and the angle read on the one quadrant only. Some years later the opposite quadrant was also graduated, to give the benefit of a second reading. The astrolabe was used by Vasco da Gama on his first voyage round the Cape of Good Hope in 1497; but the movement of a ship rendered accuracy impossible, and the liability to error was increased by the necessity for three observers. One held the instrument by a ring passed over the thumb, the second measured the altitude, and the third read off.
 |
| Fig. 2. |
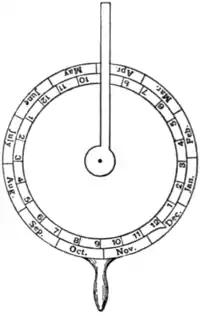
For finding latitude at night by altitude of the pole star taken by cross-staff or astrolabe, use was made of an auxiliary instrument called the “nocturnal.” From the relative positions of the two stars in the constellation of the “Little Bear” farthest from the pole (known as the Fore and Hind guards) the position of the pole star with regard to the pole could be inferred, and tables were drawn up termed the “Regiment of the Pole Star,” showing for eight positions of the guards how much should be added or subtracted from the altitude of the pole star; thus, “when the guards are in the N.W. bearing from each other north and south add half a degree,” &c. The bearings of the guards, and also roughly the hour of the night, were found by the nocturnal, first described by M. Coignet in 1581.
The nocturnal (fig. 3) consisted of two concentric circular plates, the outer being about 3 in. in diameter, and divided into twelve equal parts corresponding to the twelve months, each being again subdivided into groups of five days. The inner circle was graduated into twenty-four equal parts, corresponding to the hours of the day, and again subdivided into quarters; the handle was fixed to the outer circle in such a way that the middle of it corresponded with the day of the month on which the guards had the same right ascension as the sun—or, in other words, crossed the meridian at noon. From the common centre of the two circles extended a long index bar, which, together with the inner circle, turned freely and independently about this centre, which was pierced with a round hole. To use the instrument, the projection at twelve hours on the inner plate was turned until it coincided with the day of the month of observation, and the instrument held with its plane roughly parallel to the equinoctial or celestial equator, the observer looking at the pole star through the hole in the centre, and turning the long central index bar until the guards were seen just touching its edge; the hour in line with this edge read off on the inner plate was, roughly, the time. Occasionally the nocturnal was constructed so as to find the time by observations of the pointers in the Great Bear.
The rough charts used by a few of the more expert navigators at the time we refer to will be more fully described later (see also Map and Geography). Nautical maps or charts first appeared in Italy at the end of the 13th century, but it is said that the first seen in England was brought by Bartholomew Columbus in 1489.
Among the earliest authors who touched upon navigation was John Werner of Nuremberg, who in 1514, in his notes upon Ptolemy’s geography, describes the cross-staff as a very ancient instrument, but says that it was only then beginning to be generally introduced among seamen. He recommends measuring the distance between the moon and a star as a means of ascertaining the longitude; but this (though developed many years after into the method technically known as “lunars”) was at this time of no practical use owing to the then imperfect knowledge of the true positions of the moon and stars and the nonexistence of instrumental means by which such distances could be measured with the necessary accuracy.
 |
| Fig. 3. |
Thirty-eight years after the discovery of America, when long voyages had become comparatively common, R. Gemma Frisius wrote upon astronomy and cosmogony, with the use of the globes. His book comprised much valuable information to mariners of that day, and was translated into French fifty years later (1582) by Claude de Bossiere. The astronomical system adopted is that of Ptolemy. The following are some of the points of interest relating to navigation. There is a good description of the sphere and its circles; the obliquity of the ecliptic is given as 23° 30′. The distance between the meridians is to be measured on the equator, allowing 15° to an hour of time; longitude is to be found by eclipses of the moon and conjunctions, and reckoned from the Fortunate Islands (Azores). Latitude should be measured from the equator, not from the ecliptic, “as Clarean says.” The use of globes is very thoroughly and correctly explained. The scale for measuring distances was placed on the equator, and 15 German leagues, or 60 Italian leagues, were to be considered equal to one degree. The Italian league was 8 stadia, or 1000 paces, therefore the degree is taken much too small. We are told that, on plane charts, mariners drew lines from various centres (i.e. compass courses), which were very useful since the virtue of the lodestone had become recognized; it must be remembered that parallel rulers were unknown, being invented by Mordente in 1584. Such a confusion of lines has been continued upon sea charts till comparatively recently. Gemma gives rules for finding the course and distance correctly, except that he treats difference of longitude as departure. For instance, if the difference of latitude and difference of longitude are equal, the course prescribed is between the two principal winds—that is, 45°). He points out that the courses thus followed are not straight lines, but curves, because they do not follow the great circle, and that distances could be more correctly measured on the globe than on charts. The tide is said to rise with the moon, high water being when it is on the meridian and 12 hours later. From a table of latitudes and longitudes a few examples are here selected, by which it appears that even latitude was much in error. The figures in brackets represent the positions according to modern tables, counting the longitude from the western extremity of St Michael. (Flores is 5° 8′ farther west.)
| Alexandria | 31° | 0′ N. | (31° | 13′) | 60° | 30′ E. | (55° | 55′) |
| Athens | 37 | 15 | (37 | 58) | 52 | 45 | (49 | 46) |
| Babylon | 35 | 0 | (32 | 32) | 79 | 0 | (70 | 25) |
| Dantzic | 54 | 30 | (54 | 21) | 44 | 15 | (44 | 38) |
| London | 52 | 3 | (51 | 31) | 19 | 15 | (25 | 54) |
| Malta | 34 | 0 | (35 | 43) | 38 | 45 | (40 | 31) |
| Rome | 41 | 50 | (41 | 54) | 36 | 20 | (38 | 30) |
The latitude of Cape Clear is given 34′ in error and the longitude 412°; the Scilly Islands are given with an error of one degree in latitude and 1° 10′ in longitude; while Madeira is placed 3° 8′ too far south and 4° 20′ too far west, and Cape St Vincent 1° 25′ too far south and 6° too far west.
In 1534 Gemma produced an “astronomical ring,” which he dedicated to the secretary of the king of Hungary. He admitted that it was not entirely his own invention, but asserted that it could accomplish all that had been said of quadrants, cylinders and astrolabes—also that it was a pretty ornament, worthy of a prince. As it displayed great ingenuity, and was followed by many similar contrivances during two centuries, a sketch with brief description is here given (fig. 4).
| An image should appear at this position in the text. If you are able to provide it, see Wikisource:Image guidelines and Help:Adding images for guidance. |
Fig. 4
The outer and principal sustaining circle EPQ represents the meridian, and is about 6 in. in diameter; Pπ, are the poles. The upper quadrant is divided into degrees. It is suspended by line cord or wire placed at the supposed latitude. The second circle EQ is fixed at right angles to the first and represents the equinoctial line. The upper side is divided into twenty four parts representing the hours from noon or midnight. On the inner side of that circle are marked the months and weeks The third ring CC is attached to the first at the poles, and revolves freely within it. On the interior are marked the months and on another side the corresponding signs of the zodiac; another is graduated in degrees. It is fitted with a groove which carries two movable sights. On the fourth side are twenty-four unequal divisions (tangents) for measuring heights. Its use is illustrated by twenty problems, showing it capable of doing roughly all that any instrument for taking angles can. Thus, to find the latitude, set the sights C, C to the place of the sun in the zodiac, and shut the circle till it corresponds with 12 o'clock. Look through the sights and alter the point of suspension till the greatest elevation is attained; that time will be noon, and the point of suspension will be the latitude. The figure is represented as slung at lat. 40°, either north or south. To find the hour of the day, the latitude and declination being known: the sights C, C being set to the declination as before, and the suspension on the latitude, turn the ring CC freely till it points to the sun, when the index opposite the equinoctial circle will indicate the time, while the meridional circle will coincide with the meridian of the place.
There is in the museum attached to the Royal Naval College at Greenwich an instrument described as Sir Francis Drake’s astrolabe. It is not an astrolabe, but may be a combination of astronomical rings as invented by Gemma with additions, probably of a later date. It has the appearance of a large gold watch, about 212 in. in diameter, and contains several parts which fall back on hinges. One is a sun-dial, the gnomon being in connexion with a graduated quadrant, by which it could be set to the latitude of the place. There are a small compass and an hour circle. It is very neat, but too small for actual use, and may be simply an ornament representing a larger instrument. There is a table of latitudes engraved inside one lid; that given for London is 51° 34′, about 3 m. too much.
Though clocks are mentioned in 1484 as recent inventions, watches were unknown till about 1530, when Gemma seized the idea of utilizing them for the purpose of ascertaining the difference of longitude between two places by a comparison between their local times at the same instant. They were too inaccurate, however, to be of practical use, and their advocate proposed to correct them by water-clocks or sand-clocks. For rough purposes of keeping time on board ship sand glasses were employed, and it is curious to note that hour and half-hour glasses were used for this purpose in the British Navy until 1839. The outer margin of the compass card was early divided into twenty four equal parts numbered as hours until the error of thus determining time by the bearings of the sun was pointed out by Davis in 1607.
In 1537 Pedro Nunez (Nonius), cosmographer to the king of Portugal, published a work on astronomy, charts and some points of navigation. He recognized the errors in plane charts, and tried to rectify them. Among many astronomical problems given is one for finding the latitude of a place by knowing the sun’s declination and altitude when on two bearings, not less than 40° apart. Gemma did a similar thing with two stars; therefore the problem now known as a “double altitude” is a very old one. It could be mechanically solved on a large globe within a degree. To Nunez has been erroneously attributed the present mode of reading the exact angle on a sextant, the scale of a barometer, &c., the credit of which is due, however to Vernier nearly a hundred years later. The mode of dividing the scale which Nunez published in 1542 was the following. The arc of a large quadrant was furnished with forty-five concentric segments, or scales, the outer graduated to 90°, the others to 89, 88, 87, &c., divisions. As the fine edge of the pointer attached to the sights passed among those numerous divisions it touched one of them, suppose the fifteenth division on the sixth scale, then the angle was 1585 of 90°=15° 52′ 56″. This was a laborious method; Tycho Brahe tried it, but abandoned it in favour of the diagonal lines then in common use and still found on all scales of equal parts.
In 1545 Pedro de Medina published Arte de navigar at Valla. dolid, dedicated to Don Philippo, prince of Spain. This appears to be the first book ever published professedly entirely on navigation. It was soon translated into French and Italian, and many years after into English by John Frampton. Though this pretentious work came out two years after the death of Copernicus, the astronomy is still that of Ptolemy. The general appearance of the chart given of the Mediterranean, Atlantic, and part of the Pacific is in its favour, but examination shows it to be very incorrect. A scale of equal parts, near the centre of the chart, extends from the equator to what is intended to represent 75° of latitude; by this scale London would be in 55° instead of 5112°, Lisbon in 3714° instead of 38° 42′. The equator is made to pass along the coast of Guinea, instead of being over four degrees farther south. The Gulf of Guinea extends 14° too far east, and Mexico is much too far west. Though there are many vertical lines on the chart at unequal distances they do not represent meridians; and there is no indication of longitude. A scale of 600 leagues is given (German leagues, fifteen to a degree). By this scale the distance between Lisbon and the city of Mexico is 1740 leagues, or 6960 miles; by the vertical scale of degrees it would be about the same; whereas the actual distance is 4820 miles. Here two great wants become apparent—a knowledge of the actual length of any arc, and the means of representing the surface of the globe on flat paper. There is a table of the sun’s declination to minutes; on June 12th and December 11th (o.s.) it was given as 23° 33′. The directions for finding the latitude by the pole star and pointers appear good. For general astronomical information the book is inferior to that of Gemma.
In 1556 Martin Cortes published at Seville Arte de navigar. He gives a good drawing of the cross-staff and astrolabe, also a table of the sun’s declination for four years (the greatest value being 23° 33′), and a calendar of saints' days. The motions of the heavens are described according to the notions then prevalent, the earth being considered as fixed. He recommends the altitude of the pole being found frequently, as the estimated distance run was imperfect. He devised an instrument whereby to tell the hour, the direction of the ship’s head, and where the sun would set. A very correct table is given of the distances between the meridians at every degree of latitude, whereby a seaman could easily reduce the difference of longitude to departure. In the rules for finding the latitude by the pole star, that star is supposed to be 5° from the pole. Martin Cortes attributes the tides entirely to the influence of the moon, and gives instructions for finding the time of high water at Cadiz, when by means of a card with the moon’s age on it, revolving within a circle showing the hours and minutes, the time of high water at any other place for which it was set would be indicated. Directions are given for making a compass similar to those then in common use, also for ascertaining and allowing for the variation. The east is here spoken of as the principal point, and marked by a cross.
The third part of Martin Cortes’s work is upon charts; he laments that wise men do not produce some that are correct, and that pilots and mariners will use plane charts which are not true. In the Mediterranean and “Channel of Flanders” the want of good charts is (he says) less inconvenient, as they do not navigate by the altitude of the pole.
As some subsequent writers have attributed to Cortes the credit of first thinking of the enlargement of the degrees of latitude on Mercator’s principle, his precise words may be cited. In making a chart, it is recommended to choose a well-known place near the centre of the intended chart, such as Cape St Vincent, which call 37°, “and from thence towards the Arctic pole the degrees increase; and from thence to the equinoctial line they go on decreasing, and from the line to the Antarctic pole increasing.” It would appear at first sight that this implied that the degrees increased in length as well as being called by a higher number, but a specimen chart in the book does not justify that conclusion. It is from 34° to 40°, and the divisions are unequal, but evidently by accident, as the highest and lowest are the longest. He states that the Spanish scale was formed by counting the Great Berling as 3° from Cape St Vincent (it is under 212°). Twenty English leagues are equal to 1712 Spanish or 25 French, and to 1° of latitude. Cortes was evidently at a loss to know the length of a degree, and consequently the circumference of the globe. The degrees of longitude are not laid down, but for a first meridian we are told to draw a vertical line “through the Azores, or nearer Spain, where the chart is less occupied.” it is impossible in such circumstances to undennand or check the longitudes assigned to places at that period. Martin Cortes’s work was held in high estimation in England for many years, and appeared in several translations. A reprint, with additions, of Richard Eden’s (1561), by John Tapp and published in 1609, gives an improved table of the sun’s declination from 1609 to 1625-the maximum value being 23° to 30′. The declinations of the principal stars, the times of their passing the meridian, and other improved tables, are given, with a very poor traverse table for eight points. The cross-staff, he said, was in most common use; but he recommends Wright’s sea quadrant.
William Cuningham published in 1559 a book called his Astronomical Glass, in which he teaches the making of charts by a central meridional line divided into equal parts, with other meridians on each side, distant at top and bottom in proportion to the departure at the highest and lowest latitude, for which purpose a table of departures is given very correctly to the third place of sexagesimals. The chart would be excellent were it not that the parallels are drawn straight instead of being curved. In another example, which shows one-fourth of the sphere, the meridians and parallels are all curved; it would be good were it not that the former are too long. The hemisphere is also shown upon a projection approaching the stereographic; but the eighteen meridians cut the equator at equal distances apart instead of being nearer together towards the primitive. He gives the drawing of an instrument like an astrolabe placed horizontally, divided into 32 points and 360 degrees, and carrying a small magnetic needle to be used as a prismatic compass, or even as a theodolite.
In 1581 Michael Coignet of Antwerp published sea charts, and also a small treatise in French, wherein he exposes the errors of Medina, and was probably the first who said that rhumb lines form spirals round the pole. He published also tables of declination of the sun and observed the gradual decrease in the obliquity of the ecliptic. He described a cross-staff with three transverse pieces, which was then in common use at sea. Coignet died in 1623.
The Dutch published charts made up as atlases as early as 1584, with a treatise on navigation as an introduction. In 1585 Roderico Zamorano, who was then lecturer at the naval college at Seville, published a concise and clearly-written compendium of navigation; he follows Cortes in the desire to obtain better charts. Andres Garcia -de Cespedes, the successor of Zamorano at Seville, published a treatise on navigation at Madrid in 1606. In 1592 Petrus Plancius published his universal map, containing the discoveries in the East and West Indies and towards the north pole. It possessed no particular merit; the degrees of latitude are equal, but the distances between the meridians are varied. He made London appear in 51° 32′ N. and long. 220, by which his first meridian should have been more than 5° east of St Michael.
For Mercator’s great improvements in charts at about this date see Map; from facsimiles of his early charts in Jomard, Les Monuments de la géographie, the following measurements have been made. A general chart in 1569 of North America, from lat. 25° to lat. 79°, is 2 ft. long north and south, and 20 in. wide. Another of the same date, from the equator to 60° south lat. is 15·8 in. long. The charts agree with each other, a slight allowance being made for remeasuring. As compared with J. Inman’s table of meridional parts, the spaces between the parallels are all too small. Between 0° and 10° the error is 8′; at 20° it is 5′; at 30°, 16′; at 40°, 39′; at 50°, 61′; at 60°, 104′; at 70°, 158′; and at 79°, 182′—that is, over three degrees upon the whole chart. As the measures are always less than the truth it is possible that Mercator was afraid to give the whole. In a chart of Sicily by Romoldus Mercator in 1589, on which two equal degrees of latitude, 56° to 38°, extend 912 in., the degree of longitude is quite correct at one-fourth from the top; the lower part is 1 m. too long. One of the north of Scotland, published in 1595, by Romoldus, measures 1012 in. from 58° 20 to 61°; the divisions are quite equal and the lines parallel; it is correct at the centre only. A map of Norway, 1595, lat. 60° to 70°=912 in., has the parallels curved and equidistant, the meridians straight converging lines; the spaces between the meridians at 60° and 70° are quite correct.
In 1594 Blundeville published a description of Mercator’s charts and globes; he confesses to not having known upon what rule the meridians were separated by Mercator, unless upon such a table as that given by Wright, whose table of meridional parts is published in the same book, also an excellent table of sines, tangents and secants—the former to seven figures, the latter to eight. These are the tables made originally by Regiomontanus and improved by Clavius.
In 1594 the celebrated navigator John Davis published a pamphlet of eighty pages, in black letter, entitled The Seaman’s Secrets, in which he proposes to give all that is necessary for sailors—not for scholars on shore. He defines three kinds of sailing: horizontal, paradoxical and great circle. His horizontal sailing consists of short voyages which may be delineated upon a plain sheet of paper. The paradoxical or cosmographical, embraces longitude, latitude and distance—the combining many horizontal courses into one “infallible and true,” i.e. what is now called traverse and Mercator’s sailings. His “paradoxical course” he describes correctly as a rhumb line which is straight on the chart and a curve on the globe. He points out the errors of the common or plane chart, and promises if spared to publish a “paradoxall chart.” It is not known whether such appeared or not, but he assisted Wright in producing his chart on what is known as Mercator’s projection a few years later. Great circle sailing on a globe is clearly described by Davis, and ta render it more practicable he divides a long distance into several short rhumb lines quite correctly. From the practice oi navigators in using globes the principles of such sailing were not unknown at an earlier date; indeed it is said that S. Cabot. projected a voyage across the North Atlantic on the arc of a great circle in 1495.
The list of instruments given by Davis as necessary to a skilful seaman comprises the sea compass, cross-staff, chart, quadrant, astrolabe, an “instrument magnetical” for finding the variation of the compass, a horizontal plane sphere, a globe and a paradoxical compass. The first three are said to be sufficient for use at sea, the astrolabe and quadrant being uncertain for sea observations. The importance of knowing the times of the tides when approaching tidal or barred harbours is clearly pointed out, also the mode of ascertaining them by the moon’s age. A table of the sun’s declination is given for noon each day during four years 1593–1597, from the ephemerides of J. Stadius. The greatest given value is 23° 28′ Several courses and distances, with the resulting difference of latitude and departure, are correctly worked out. A specimen log-book provides one line only for each day, but the columns are arranged similarly to those of a modern log. Under the head of remarks after leaving Brazil, we read, “the compass varied 9°, the south point westward.” He states that the first meridian passed through St Michael, because there was no variation at that place, and therefore that this meridian passed through the magnetic pole as well as the pole of the earth. He makes no mention of Mercator’s chart by name nor of Cortes or other writers on navigation. Rules are given for finding the latitude by two altitudes of the sun and intermediate azimuth, also by two fixed stars, using a globe. There is a drawing of a quadrant, with a plumb line, for measuring the zenith distance, and one of a modification of a cross-staff using which the observer stands with his back to the sun, looking at the horizon through a sight on the end of the staff, while the shadow of the top of a movable projection, falls on the sight; this, known as the back-staff, was an improvement on the cross-staff. It was fitted with a reflector, and was thus the first rough idea of the principle of the quadrant and sextant. This remained in common use till superseded in 1731 by Hadley’s quadrant. The eighth edition of Davis’s work was printed in 1657.
Edward Wright, of Caius College, Cambridge, published in 1599 a valuable work entitled Certain Errors in Navigation Detected and Corrected. One part is a translation from Roderico Zamorano; there is a chapter from Cortes and one from Nunez. A year later appeared his chart of the world, upon which both capes and the recent discoveries in the East Indies and America are laid down truthfully and scientifically, as well as his knowledge of their latitudes and longitudes would admit. Just the northern extremity of Australia is shown.
Wright said of himself that he had striven beyond his ability to mend the errors in chart, compass, cross-staff and declination of sun and stars. He considered that the instruments which had then recently come in use “could hardly be amended,” as they were growing to “perfection”—especially the sea chart and the compass, though he expresses a hope that the latter may be “ freed from that rude and gross manner of handling in the making.” He gives a table of magnetic declinations (variation) and explains its geometrical construction. He states that Medina utterly denied the existence of variation, and attributed it to bad construction and bad observations. Wright expresses a hope that a right understanding of the dip of the needle would lead to a knowledge of the latitude, “as the variation did of the longitude.” He gives a table of declination of the sun for the use of English mariners during four years-the greatest given value being 23° 31′ 30″. The latitude of London he made 51° 32′. For these determinations a quadrant over 6 ft. in radius was used. He also treats of the “dip” of the sea horizon, refraction, parallax and the sun’s motions. With all this knowledge the earth is still considered as stationary-although Wright alludes to Copernicus, and says that he omitted to allow for parallax. Wright ascertained the declinations of thirty-two stars, and made many improvements or additions to the art of navigation, considering that all the problems could be performed trigonometric ally, without globe or chart. He devised sea rings for taking observations, and a sea quadrant to be used by two persons, which is in some respects similar to that by Davis. While deploring the neglected state which navigation had been in, he rejoices that the worshipful society at the Trinity House (which had been established in 1514), under the favour of the king (Henry VIII.), had removed “many gross and dangerous enormities.” He joins the brethren of the Trinity House in the desire that a lectureship should be established on navigation, as at Seville and Cadiz; also that a grand pilot should be appointed, as Sebastian Cabot had been in Spain, to examine pilots (i.e. mates) and navigators. Wright’s desire was partially fulfilled in 1845, when an Act of Parliament paved the way for the compulsory qualification of masters and mates of merchant ships; but such was the opposition by shipowners that it was even then left voluntary for a few years. England was in this respect more than a century behind Holland. It has been said that Wri ht accompanied the earl of Cumberland to the Azores in 1589, and that he was allowed £50 a year by the East éndia Company as lecturer on navigation at Gresham College, Tower Street.
The great mark which Wright made was the discovery of a correct and uniform method of dividing the meridional line and making charts which are still called after the name of Mercator. He considered such charts as true as the globe itself; and so they were for all practical purposes. He commenced by dividing a meridional line, in the proportion of the secants of the latitude, for every ten minutes of arc, and in the edition of his work published in IOIO his calculations are for every minute. His method was based upon the fact that the radius bears the same proportion to the secant of the latitude as the difference of longitude does to the meridional difference of latitude-a rule strictly correct for small arcs only. One minute is taken as the unit upon the arc and 10,000 as the corresponding secant, 2′ becomes 20,000, 3′=30,000, &c., increasing uniformly till 49′, which is equal to 490,001; 1° is 600,012. The secant of 20° is 12,251,192, and for 20° 1′ it will be 12,251,192+10,642—practically the same as that used in modern tables.
The principle is simply explained by fig. 5, where b is the pole and bf the meridian. At any point a a minute of longitude: a min. of lat.: : ea (the semi-diameter of the parallel): kf (the radius). Again ea: kf: :kf:ki: :radius :sec. akf (sec. of lat). To keep this proportion on the chart, the distances between points of latitude must increase in the same proportion as the secants of the arc contained between those points and the equator, which was then to be done by the “canon of triangles.”
| An image should appear at this position in the text. If you are able to provide it, see Wikisource:Image guidelines and Help:Adding images for guidance. |
Wright gave the following excellent popular description of the principle of Mercator’s charts; “Suppose a spherical globe (representing the world) inscribed in a concave cylinder to swell like a bladder equally in every part (that is as much in longitude as in latitude) until it Joins itself to the concave surface of the cylinder, each parallel increasing successively from the equator towards either pole until it is of equal diameter to the cylinder, and consequently the meridians widening apart until they are everywhere as distant from each other as they are at the equator. Such a spherical surface is thus by extension made cylindrical, and consequently a plane parallelogram m surface, since the surface of a cylinder is nothing else but a plane parallelogram surface wound round it. Such a cylinder on being opened into a flat surface will have upon it a representation of a Mercator’s chart of the world.”
This great improvement in the principle of constructing charts was adopted slowly by seamen, who, putting it as they supposed to a practical test, found good reason to be disappointed. The positions of most places in the world had been originally laid down erroneously, by very rough courses and estimated distances upon the plane chart, and from this they were transferred to the new projection, so that errors in courses and distances, really due to erroneous positions, were wrongly attributed to the new and accurate form of chart.
When Napier’s Canon Mirificus appeared in 1614, Wright at once recognized the value of logarithms as an aid to navigation, and undertook a translation of the book, which he did not live to publish (see Napier). Gunter’s tables (1620) made the application of the new discovery to navigation possible, and this was done by Addison in his Arithmetical Navigation (1625), as Well as by Gunter in his tables of 1624 and 1636, which gave logarithmic sines and tangents, to a radius of 1,000,000, with directions for their use and application to astronomy and navigation, and also logarithms of numbers from 1 to 10,000. Several editions followed, and the work retained its reputation over a century. Gunter invented the sector, and introduced the meridional line upon it, in the just proportion of Mercator’s projection. The means of taking observations correctly, either at sea or on shore, was about this time greatly assisted by the invention bearing the name of Pierre Vernier, the description of which was published at Brussels in 1631. As Vernier’s quadrant was divided into half degrees only, the sector, as he called it, spread over 14% degrees, and that space carried thirty equal divisions, numbered from 0 to 30. As each division of the sector contained 29 min. of arc, the vernier could be read to minutes. The verniers now commonly adapted to sextants can be read to 10 secs. Shortly after the invention it was recommended for use by P. Bouguer and Jorge Juan, who describe it in a treatise entitled La Construction, &c., du quadrant nouveau. About this period Gascoigne applied the telescope to the quadrant as used on shore; and Hevelius invented the tangent screw, to give slow and steady motion when near the desired position. These practical improvements were not applied to the rougher nautical instruments until the invention of Hadley's sextant in 1731.
In 1635 Henry Gellibrand published his discovery of the annual change in variation of the needle, which was effected by comparing the results of his own observations with those of W. Borough and Edmund Gunter. The latter was his predecessor at Gresham College.
In 1637 Richard Norwood, a sailor, and reader in mathematics, published an account of his most laudable exertions to remove one of the greatest stumbling-blocks in the way of correct navigation, that of not knowing the true length of a degree or nautical mile, in a pamphlet styled The Seaman’s Practices. Norwood ascertained the latitude of a position near the Tower of London in June 1633, and of a place in the centre of York in June 1635, with a sextant of more than 5 ft. radius, and, having carefully corrected the declination of the sun and allowed for refraction and parallax, made the difference of latitude 2° 28′. He then measured the distance with a chain, taking horizontal angles of all windings, and made a special table for correcting elevations and depressions. A few places which he was unable to measure he paced. His conclusion was that a degree contained 367,176 English feet; this gives 2040 yds. to a nautical mile—only about 12 yds. too much. Norwood’s work went through numerous editions, and retained its popularity over a hundred years. In a late edition he says that, as there is no means of discovering the longitude, a seaman must trust to his reckoning. He recommends the knots on the log-line to be placed 51 ft. apart, as the just proportion to a mile when used with the half-minute glass. To Norwood is also attributed the discovery of the “dip” of the magnetic needle in 1576.
The progress of the art of navigation was and is still of course inseparably connected with that of map and chart drawing and the correct astronomical determinations of positions on land. While as we have seen at an early period simple practical astronomical means of finding the latitude at sea were known and in use, no mode could be devised of finding longitude except by the rough method of estimating the run of the ship, so that the only mode of arriving at a port of destination was to steer so as to get into the latitude of such a port either to the eastward or westward of its supposed position, and then approach it on the parallel of its latitude. The success of this method would of course greatly depend upon the accuracy with which the longitude of such port was known. Even with the larger and more accurate instruments used in astronomical observatories on shore the means of ascertaining latitude were far in advance of those by which longitude could be obtained, and this equally applied to the various heavenly bodies themselves upon which the terrestrial positions depended, the astronomical element of declination (corresponding to latitude) being far more accurately determined than that of right ascension (corresponding to longitude).
Almanacs were first published on the continent of Europe in 1457, but the earliest printed work of that kind in England is dated 1497. The only portions of their contents of use to seamen were tables of the declination of the sun, rough elements of the positions of a few stars, and tables for finding latitude by the pole star.
No accurate predictions of the positions of the moon, stars and planets could, however, be made until the laws governing their movements were known, such laws of course involving a knowledge of their actual positions at different widely separated epochs.
In 1699 Edmund Halley (subsequently astronomer royal), in command of the “Paramour,” undertook a voyage to improve the knowledge of longitude and of the variation of the compass. The results of his voyage were the construction of the first variation chart, and proposals for finding the longitude by occultations of fixed stars.
The necessity for having more correct charts being equalled by the pressing need of obtaining the longitude by some simple and correct means available to seamen, many plans had already been thought of for this purpose. At one time it was hoped that the longitude might be directly discovered by observing the variation of the compass and comparing it with that laid down on charts. In 1674 Charles II. actually appointed a commission to investigate the pretensions of a scheme of this sort devised by Henry Bond, and the same idea appears as late as 1777 in S. Dunn’s Epitome. But the only accurate method of ascertaining the longitude is by knowing the difference of time at the same instant at the meridian of the observer and that of Greenwich; and till the invention and perfecting of chronometers this could only be done by finding at two such places the apparent time of the same celestial phenomenon.
A class of phenomena whose comparative frequency recommended them for longitude observations, viz. the eclipses of Jupiter’s satellites, became known through Galileo’s discovery of these bodies (1610). Tables for such eclipses were published by Dominic Cassini at Bologna in 1688, and repeated in a more correct form at Paris in 1693 by his son, who was followed by J. Pound, J. Bradley, P. W. Wargentin, and many other astronomers. But this method, though useful on land, is not suited to mariners; when W. Whiston, for example, in 1737 recommended that the satellites should be observed by a reflecting telescope, he did not sufficiently consider the difficulty of using a telescope at sea.
Another method proposed was that of comparing the local time of the moon’s crossing the meridian of the observer with the predicted time of the same event at Greenwich, the difference of the two depending upon the moon’s motion during the time represented by the longitude; thus Herne’s Longitude Unveiled (1678), proposes to find the time of the moon’s meridian passage at sea by equal altitudes with the cross-staff, and then compare apparent time at ship with London time. The accuracy of this, as in the case of lunar problems, would obviously depend upon a more perfect knowledge of the laws of the moon’s motion than then existed.
The celebrated problem of finding longitude by lunars (or by measurement of “lunar distances”) occupied the attention of astronomers and sailors for many years before being superseded by the more simple and accurate modern method by the use of chronometers, and was the principal reason for establishing the Royal Observatory at Greenwich and the subsequent publication of the Nautical Almanac. The principle was simple, depending upon the comparatively rapid movement of the moon with regard to the heavenly bodies lying in her immediate path in the heavens. It is evident that if the theory of this movement were perfectly understood and the positions of such heavenly bodies accurately determined, the distances of the moon from those at any instant of time at Greenwich could be accurately foretold so that if such predictions were published in advance, an observer at any place in the world, by simply measuring such distances, could accurately determine the Greenwich time, a comparison of which with the local time (which in clear weather can be frequently and simply determined) would give the longitude. This, as previously mentioned, was foreseen by J. Werner as early as 1514, but very great difficulties attended its practical application for many years. Until the establishment of national astronomical observatories it was impossible to accumulate the vast number of observations necessary to fufil the astronomical conditions, and until the invention of the sextant no instrument existed capable of use at sea which would measure the distances required with the necessary accuracy, while even up to the time when the problem had attained its greatest practical accuracy the calculations involved were far too intricate for general use among those for whom it was chiefly intended. The very principles of a theory of the movements of the moon were unknown before Newton’s time, when the lunar problem begins to have a chief place in the history of navigation; the places of stars were formerly derived from various and widely discrepant sources.
The study of the lunar problem was stimulated by the reward of 1000 crowns offered by Philip III. of Spain in 1598 for the discovery of a method of finding longitude at sea; the States-general followed with an offer of 10,000 florins. But for a long time nothing practical came of this; a. proposal by J. B. Morin, submitted to Richelieu in 1633, was pronounced by commissioners appointed to judge of it to be impracticable through the imperfection of the lunar tables, and the same objection applied when the question was raised in England in 1674 by a proposal of St Pierre to find the longitude by using the altitudes of the moon and two stars to find the time each was from the meridian. When the king was pressed by St Pierre, Sir J. Moore and Sir C. Wren to establish an observatory for the benefit of navigation, and especially that the moon’s exact position might be calculated a year in advance, Flamsteed gave his judgment that the lunar tables then in use were quite useless, and the positions of the stars erroneous. The result was that the king decided upon establishing an observatory in Greenwich Park, and Flamsteed was appointed astronomical observer on March 4, 1675, upon a salary of £100 a year, for which also he was to instruct two boys from Christ’s Hospital. While the small building in the Park was in course of erection he resided in the Queen’s House (now the central part of Greenwich Hospital school), and removed to the house on the hill on the 10th of July 1676, which came to be known as “Flamsteed House.” The institution was placed under the surveyor-general of ordnance—perhaps because that office was then held by Sir Jonas Moore, himself an eminent mathematician. Though this was not the first observatory in Europe, it was destined to become the most useful, and has amply fulfilled the important duties for which it was designed. It was established to meet the exigencies of navigation, as was clearly stated on the appointment of Flamsteed, and on several subsequent occasions; we see now what an excellent foster-mother it has been to the higher branches of that science. This has been accomplished by much labour and patience; for, though originally the most suitable man in the kingdom was placed in charge, it was so starved and neglected as to be almost useless during many years. The government did not provide a single instrument. Flamsteed entered upon his important duties with an iron sextant of 7 ft. radius, a quadrant of 3 ft. radius, two telescopes and two clocks, the last given by Sir Jonas Moore. Tycho Brahe’s catalogue of 777 stars, formed in about 1590, was his only guide. In 1681 he fitted a mural arc which proved a failure. Seven years after another mural arc was erected at a cost of £120, with which he set to work in earnest to verify the latitude, and to determine the position of the equinoctial point, the obliquity of the ecliptic and the right ascensions and declinations of the stars; he obtained the positions of 2884 which appeared in the “British catalogue” in 1723 (see Flamsteed, and Astronomy).
Flamsteed died in 1719, and was succeeded by Halley, who paid particular attention to the motions of the moon with a view to the longitude problem. A paper which he published in the Phil. Trans. (1731) shows what had been accomplished up to that date, and proves that it was still impossible to find the longitude correctly by any observation depending upon the predicted position of the moon. He repeats what he had published twenty years before in an appendix to Thomas Street’s Caroline tables, which contained observations made by him (Halley) in 1683–1684 for ascertaining the moon’s mot1on, which he thought to be the only practical method of “attaining” the longitude at sea. The Caroline tables of Street, though better than those before his time as well as those of Tycho, Kepler, Bullialdus and Horrox, were uncertain; sometimes the errors would compensate one another; at others when they fell the same way the result might lead to a position being 100 leagues in error. He hopes that the tables will be so amended that an error may scarce ever exceed 3 minutes of arc (equal to 112° of longitude). Sir Isaac Newton’s tables, corrected by himself (Halley) and others up to 1713, would admit of errors of 5 minutes, when the moon was in the third and fourth quarters. He blames Flamsteed for neglecting that portion of astronomical work, as he was at the observatory more than two periods of eighteen years. He himself had at this time seen the whole period of the moon’s apogee—less than nine years—during which he observed the right ascensions at her transit, with great exactness, almost fifteen hundred times, or as often as Tycho Brahe, Hevelius and Flamsteed together. He hoped to be able to compute the moon’s position within 2 minutes of arc with certainty, which would reduce errors of position to 20 leagues at the equator and 15 in the Channel; he thought Hadley’s quadrant might be applied to measure lunar distances at sea with the desired accuracy.[1]
The rise of modern navigation may be fairly dated from the invention of the sextant in 1731 and of the chronometer in 1735; the former a complete nautical observatory in itself, and the latter an instrument which in its modern development has become an almost perfect time-keeper. It was a curious coincidence that these two invaluable instruments were invented at so nearly the same time. Until 1731 all instruments in use at sea for measuring angles either depended on a plumb line or required the observer to look in two directions at once.
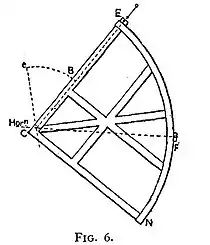
Their imperfections are clearly pointed out in a paper by Pierre Bouguer (1729) which received the prize of the Paris Academy of Sciences for the best method of taking the altitude of stars at sea. Bouguer himself proposes a modification of what he calls the English quadrant, probably the one suggested by Wright and improved by Davis. Fig. 6 represents the instrument as proposed, capable of measuring fully 90° from E to N. A fixed pinule was recommended to be placed at E, through which a ray from the sun would pass to the sight C. The sight F was movable. The observer, standing with his back to the sun would look through F and C at the horizon, shifting the sight F up or down till the ray from the sun coincided with the horizon. The space from E to F would represent the altitude, and the remaining part F to N the zenith distance. The English quadrant which this was to supersede differed in having about half the arc from E towards N, and, instead of the pinule being fixed at E, it was on a smaller arc represented by the dotted line eB, and movable. It was placed on an even number of degrees, considerably less than the altitude; the remainder was measured on the larger arc, as described.
Hadley’s instrument, on the other hand, described to the
Royal Society in May 1731 (Phil. Trans.), embodies Newton’s
idea of bringing the reflection of one object to coincide with the
direct image of the other. He calls it an octant, as the arc is
actually 45°, or the eighth part of a circle; but, in consequence
 of the angles of incidence and
reflection both being changed
by a movement of the index.
it measures an angle of 90°, and
is graduated accordingly; the
same instrument has therefore
been called a quadrant. It was
very slowly adopted, and no
doubt there were numerous
mechanical difficulties of centring,
graduating, &c., to be
overcome before it reached perfection.
In August 1732, in
pursuance of an order from the
Admiralty, observations were
made with Hadley’s quadrant
on board the “Chatham”
yacht of 60 tons, below Sheerness,
in rough weather, by persons—except the master attendant—unaccustomed
to the motion; still the results were very satisfactory.
A year later Hadley published (Phil. Trans., 1733) the
description of an instrument for taking altitudes when the
horizon is not visible. The sketch represents a curved tube or
spirit-level, attached to the radius of the quadrant, since which
time many attempts have been unsuccessfully made to construct
some form of artificial horizon adapted to use at sea on board
ship, a discovery which would greatly facilitate observations at
night and at the many times when the natural or sea horizon is
imperfectly visible.
of the angles of incidence and
reflection both being changed
by a movement of the index.
it measures an angle of 90°, and
is graduated accordingly; the
same instrument has therefore
been called a quadrant. It was
very slowly adopted, and no
doubt there were numerous
mechanical difficulties of centring,
graduating, &c., to be
overcome before it reached perfection.
In August 1732, in
pursuance of an order from the
Admiralty, observations were
made with Hadley’s quadrant
on board the “Chatham”
yacht of 60 tons, below Sheerness,
in rough weather, by persons—except the master attendant—unaccustomed
to the motion; still the results were very satisfactory.
A year later Hadley published (Phil. Trans., 1733) the
description of an instrument for taking altitudes when the
horizon is not visible. The sketch represents a curved tube or
spirit-level, attached to the radius of the quadrant, since which
time many attempts have been unsuccessfully made to construct
some form of artificial horizon adapted to use at sea on board
ship, a discovery which would greatly facilitate observations at
night and at the many times when the natural or sea horizon is
imperfectly visible.
From the year 1714 the history of navigation in England is closely associated with that of the “Commissioners for the discovery of longitude at sea,” a body constituted in that year with power to grant annually sums not exceeding £2000 to assist experiments and reward minor discoveries. and also to judge on applications for much greater rewards which were from time to time offered to open competition. For a method of determining the longitude within 60 geographical miles, to be tested by a voyage to the West Indies and back, the sum of £10,000 was offered; within 40 m., £15,000; within 30 m., £20,000. £10,000 was also to be given for a method that would determine longitude within 80 m. near the shores of greatest danger. No action seems to have been taken before 1737; the first grant made was in that year, and the last in 1815, but the board continued to exist till 1828, having disbursed in the course of its existence £101,000 in all.[2] In the interval a number of other acts had been passed either dealing with the powers, constitution and funds of the commissioners or encouraging nautical discovery; thus the act 18 George II. (1745) offered £20,000 for the discovery by a British ship of the North-West Passage, and the act 16 George III. (1776) offered the same reward for a passage to the Pacific either north-west or north-east, and £5000 to any one who should approach by sea within one degree of the North Pole. All these acts were swept away in 1828, when the longitude problem had ceased to attract competitors, and voyages of discovery were nearly over.
The suggestions and applications sent in to the commissioners were naturally very numerous and often very trifling; but they sometimes furnish useful illustrations of the state of navigation. Thus, in a memorial by Captain H. Lanoue (1736), he records a number of recent casualties, which shows how carelessly the largest ships were then navigated. Several men-of-war off Plymouth in 1691 were wrecked through mistaking the Deadman for Berry Head. Admiral Wheeler’s squadron in 1694, leaving the Mediterranean, ran on Gibraltar when they thought they had passed the Strait. Sir Cloudesley Shovel’s squadron, in 1707, was lost on the rocks off Scilly, by erring in their latitude. Several transports, in 1711, were lost near the river St Lawrence, having erred 15 leagues in the reckoning during twenty-four-hours. Lord Belhaven was lost on the Lizard on the 17th of November 1721, the same day on which he sailed from Plymouth.
Many rewards were paid by the commissioners for methods by which the tedious calculations involved in “clearing the lunar distance” could be abbreviated; thus Israel Lyons (1739–1775) received £10 for his solution of this problem from the commissioners in 1769; and in 1772 he and Richard Dunthorne (1711–1775) each obtained £50. George Whichell, master of the Royal Naval Academy, Portsmouth, conceived a plan whereby the correction could be taken from a table by inspection. In October 1765 the commissioners of longitude awarded him £100 to enable him to complete and print 1000 copies of his table. On the following April they gave him £200 more. The work was continued on the same plan by Antony Shepherd, the Plumian professor of astronomy, Cambridge, with some additions by the astronomer-royal. The total cost of the ponderous 4to volume up to the time of publication in June 1772 was £3100, after which £200 more was paid to the Rev. Thomas Parkinson and Israel Lyons for examining the errata. It was a very large and expensive volume-ill-adapted for ship’s use. Considerable sums were paid by the commissioners from time to time for other tables to facilitate navigation-not always very judiciously. It is sufficient to mention here the tables of Michael Taylor and those of Mendoza, published in ISIS. The proposals submitted to the board to find the longitude by the time of the moon’s meridian passage are very numerous.
One of the first points to which the attention of the commissioners was directed was the survey of the coasts of Great Britain, which was pressed on them by Whiston in 1737. He was appointed surveyor of coasts and headlands, and in 1741 received a grant for instruments. An act passed in 1740 enabled the commissioners to spend money on the survey of the coasts of Great Britain and the “plantations.” At a later date they bore part of the expenses of Cook’s scientific voyages, and of the publication of their results. Indeed it is to them that we owe all that was done by England for surveys of coasts, both at home and abroad, prior to the establishment of the hydrographic department of the Admiralty in 1795. But their chief work lay in the encouragement they gave on the one hand to the improvement of timepieces, and on the other to the perfecting of astronomical tables and methods, the latter being published from time to time in the Nautical Almanac. Before we pass on to these two important topics we may with advantage take a view of the state of practical navigation in the middle of the 18th century as shown in two of the principal treatises then current.
John Robertson’s Elements of Navigation passed through six editions between 1755 and 1796. It contains good teaching on arithmetic, geometry, spherical trigonometry, astronomy, geography, winds and tides, also a small useful table for correcting the middle time between the equal altitudes of the sun-all good, as is also the remark that “the greater the moon’s meridian altitude the greater generally the tides will be.” He states that Lacaille recommends equal altitudes being observed and worked separately, 'in order to find the time from noon, and the mean of the results taken as the truth. There is a sound article on chronology, the ancient and modern modes of reckoning time. A long list of latitudes, longitudes and times of high water finishes vol. i. The second volume is said by the author to treat of navigation mechanical and theoretical; by the former he means seamanship. He gives instructions for all kinds of sailings, for marine surveying and making Mercator’s chart. There are two good traverse tables, one to quarter points, the other to every 15 minutes of arc; the distance to each is 120 m. There is a table of meridional parts to minutes, which is more minute than customary. Book ix., upon what is now called “the day’s work,” or dead-reckoning, appears to embrace all that is necessary. A great many methods, we are told, were then used for measuring a ship’s rate of sailing, but among the English the log and line with a half-minute glass were generally used. Bouguer and Lacaille proposed a log with a diver to avoid the drift motion (1753 and 1760). Robertson’s rule of computing the equation of equal altitudes is as good as any used at the present day. He gives also a description of an equal-altitude instrument, having three horizontal wires, probably such as was used at Portsmouth for testing Harrison’s timekeeper. The mechanical difficulties must have been great in preserving a perpendicular stem and a truly horizontal sweep for the telescope. It gave place to the improved sextant and artificial horizon. The second edition of Robertson’s work in 1764 contains an excellent dissertation on the rise and progress of modern navigation by Dr James Wilson, which has been greatly used by all subsequent writers.
Don Jorge Juan’s Compendio de Navegacion, for the use of midshipmen, was published at Cadiz in 1757. Chapter i. explains what pilotage is, practical and theoretical. He speaks of the change of variation, “which sailors have not believed and do not believe now.” He describes the lead, log and sand-glass, the latter corrected by a pendulum, charts plane and spherical. Supposing his readers to be versed in trigonometry, he explains what latitude and longitude are, and shows a method for finding the latter different from what has been taught. He explains the error of middle latitude sailing, and shows that the longitude found by it is always less than the truth. (It is strange that while reckoning was so rough and imperfect in many respects such a trifle as that is in low latitudes should be noticed.) After speaking of meridional parts, he offers to explain the English method, which was discovered by Edmund Halley, but omits the principles upon which Halley founded his theory, as it was “too embarrassing.” He gives instructions for allowing for currents and leeway, tables of declination, positions of a few stars, meridional parts, &c. It is worthy of remark that, after giving a form for a log-book, he adds that this had not been previously kept by any one, but he thought it should not be trusted to memory. He only requires the knots, fathoms, course, wind and leeway to be marked every two hours. He gives a sketch of Halley’s quadrant, but without a clamping screw or tangent screw.
To ascertain local time at sea by astronomical observations by the altitude of suitably-situated heavenly bodies was an old, well-known and frequently practised operation, so that a comparison could thus be easily made between such local time and the Greenwich time if known at the same instant. The introduction of timekeepers by which Greenwich time can be carried to any part of the world, and the longitude found with ease, simplicity and certainty is due to the invention of John Harrison.
The idea of keeping time at sea by watches was no novelty, but the practical difficulty arose from their very irregular rates owing to changes of temperature and the motion of the ship. Huygens had applied pendulums to the regulation of clocks on shore in 1656, and in 1675 his application of spiral springs as regulators of watches made them available for use at sea. William Derham published a scientific description of various kinds of timekeepers in The Artificial Clock-Maker, in 1700, with a table of equations from Flamsteed to facilitate comparison of mean time with that shown by the sfun-dial or apparent time. In 1714 Henry Sully, an Englishman, published a treatise at Vienna, on finding time artificially. He went to France, and spent the rest of his life in trying to make a timekeeper for the discovery of the longitude at sea. In 1716 he presented a watch of his own make to the Academy of Sciences, which was approved; and ten years later he went to Bordeaux to try his marine watches, but died before embarking. Julien le Roy was his scholar, and perfected many of his inventions in watchmaking.
Harrison’s great invention was the principle of compensation through the unequal contraction of two metals, which he first applied in the invention in 1726 of the compensation (gridiron) pendulum, still in use, and then modified so as to fit it to a watch, devising at the same time a means by which the watch retains its motion while being wound up. With regard to the success of the trial journey (see Harrison, John) to Jamaica in 1761–1762, it may be noted that by the journal of the House of Commons we find that the error of the watch was ascertained by equal altitudes at Portsmouth and Barbados, the calculations being made by Short; these errors came greatly within the limits of the act. At Jamaica the watch was only in error five seconds (assuming that the longitude previously found by the transit of Mercury could be closely depended) on, which as we now know, was not the case, the observations being too few in number, and taken with an untrustworthy instrument). Short at Portsmouth found the whole unhallowed-for error from November 6th, 1761, till April 2nd, 1762, to be 1m54″.5 = 18 geographical miles in the latitude of Portsmouth. During the passage home in the “Merlin” sloop-of-war the timekeeper was placed in the after part of the ship, because it was the dryest place, and there it received violent shocks which retarded its motion. It lost on the voyage home 1m 49″ = 16 geographical miles.
One might have supposed that Harrison had now secured the prize; but there were powerful competitors who hoped to gain it by lunars, and a bill was passed through the House in 1763 which left an open chance for a lunarian during four years. A second West Indies trial of the watch took place between November 1763 and March 1764, in a voyage to Barbados, which occupied four months; during which time it is said, in the preamble to act 5 Geo. III. 1765, not to have erred 10 geographical miles in longitude. We only find in the public records the equal altitudes taken at Portsmouth and at Bridgetown, Barbados. William Harrison assumed an average rate of 1″ a-day gaining, and he anticipated that it would go slower by 1″ for every 10° increase in temperature. The longitude of Bridgetown was determined by N. Maskelyne and C. Green by nine emersions of Jupiter’s first satellite, against five of Bradley's and two at Greenwich Observatory, to be 3h 54m 20s west of Greenwich. In February 1765 the commissioners of longitude expressed an opinion that the trial was satisfactory, but required the principles to be disclosed and other watches made. Half the great reward was paid to Harrison under act of parliament in this year, and he and his son gave full descriptions and drawings, upon oath, to seven persons appointed by the commissioners of longitude.[3] The other half of the great reward was promised to Harrison when he had made other timekeepers to the satisfaction of the commissioners, and provided he gave up everything to them within six months. The second half was not paid till 1773, after trials had been made with five watches. These trials were partly made at Greenwich by Maskelyne, who, as we shall see, was a great advocate of lunars, and was not ready to admit more than a subsidiary value to the watch. A bitter controversy arose, and Harrison in 1767 published book in which he charges Maskelyne with exposing his watch to unfair treatment. The feud between the astronomer-royal and the watchmakers continued long after this date.
Even after Harrison had received his £20,000, doubts were felt as to the certainty of his achievement, and fresh rewards were offered in~1774 both for timekeepers and for improved lunar tables or other methods. But the tests proposed for timekeepers were very discouraging, and the watchmakers complained that this was due to Maskelyne. A fierce attack on the astronomer’s treatment of himself and other watchmakers was made by Thomas Mudge in 1792, in A Narrative of Facts, addressed to the first lord of the Admiralty, and Maskelyne’s reply does not convey the conviction that full justice was done to timekeepers. Maskelyne at this date still says that he would prefer an occultation of a bright star by the moon and a number of correspondent observations of transits of the moon compared with those of fixed stars, made by two astronomers at remote places, to any timekeeper. The details of these controversies, and of subsequent improvements in timekeepers, need not detain us here. In England the names of John Arnold and Thomas Earnshaw as watchmakers are prominent, each of whom received, up to 1805, £3000 reward from the commissioners of longitude. It was Arnold who introduced the name chronometer. The French emulated the English efforts for the production of good timekeepers, and favourable trials were made between 1768 and 1772 with watches by Le Roy and F. Berthoud.
The marvellous accuracy with which the modern chronometer is constructed is doubtless greatly stimulated by the annual competition at Greenwich, from which the Admiralty purchase for the British navy. These chronometers are all fitted with secondary compensation balances, and it is therefore unusual in the navy to apply any temperature correction to the rate. The perfection obtainable in compensation may be illustrated by the performance of a chronometer at the Royal Observatory in 1886, which at a mean temperature of 50° F. had a weekly rate of 1·6 secs. losing; and on being further tested at a mean temperature of 92° F., it only changed its weekly rate to 2·9 secs. losing. In the mercantile marine cheaper chronometers without secondary compensation are more commonly used, and temperature corrections applied, calculated from a formula originally proposed by Hartnup, formerly of the Liverpool Observatory. Great success attends this mode of procedure, as illustrated by the following facts. From the discussion of the records of performance of the chronometers of the Pacific Steam Navigation Company during twenty-six voyages from London to Valparaiso and back, by giving equal weight to each of the three chronometers carried by each ship, the mean error of longitude for an average voyage of 101 days was less than three minutes of arc. As a single instance, in the s.s. Orellana, on applying temperature rates during a voyage of 63 days, the mean accumulated error of the three chronometers was only 2·3 sec. of time.
While chronometers were thus rapidly approaching their present perfection the steady progress of astronomy both by the multiplication and increased accuracy of observations, and by corresponding advances in the theory, had made it possible to construct greatly improved tables. In observations of the moon Greenwich still took the lead; and it was here that Halley’s successor Bradley made his two grand discoveries of aberration and nutation which have added so much to the precision of modern astronomy. Kepler’s Rudolphine tables of 1627 and Street’s tables of 1661, which had held their ground for almost a century, were rendered obsolete by the observations of Halley and his successor. At length, in 1753, in the second volume of the Commentarii of the Academy of Göttingen, Tobias Mayer printed his new solar and lunar tables, which were to have so great an influence on the history of navigation. Mayer afterwards constructed and submitted to the English government in 1755 improved MS. tables. Bradley found that the moon’s place by these tables was generally correct within 1′, so that the error in a longitude found by lunar would not be much more than half a degree if the necessary observations could be taken accurately at sea. Thus the lunar problem seemed to have at length become a practical one for mariners, and in England it was taken up with great energy by Nevil Maskelyne—“the father,” as he has been called, “of lunar observations.”
In 1761 Maskelyne was sent to St Helena to observe the transit of Venus. On his voyage out and home he used Mayer’s printed tables for lunar determinations of the longitude, and from St Helena he wrote a letter to the Royal Society (Phil. Trans., 1762), in which he described his observations made with Hadley’s quadrant of 20 in. radius, constructed by John Bird, and the glasses ground by Dollond. He took the observations both ways to avoid errors. The arc and index were of brass, the frame mahogany; the vernier was subdivided to minutes. The telescope was 6 in. long, magnified four times, and inverted. Very few seamen in that day possessed so good an instrument. He considered that ship’s time should be ascertained within twelve hours before or after observing the lunar distance, as a good common watch will scarcely vary above a minute in that time. This shows that he must have intended the altitudes to be calculated—which would lead to new errors. He considered that his observations would give the longitude within 112 degrees. On the 11th of February he took ten observations; the extremes were a little over one degree apart.
On his return to England Maskelyne prepared the British Mariner’s Guide (1763), in which he undertakes to furnish complete and easy instructions for finding the longitude at sea or on shore, within a degree, by observing the distance between the moon and sun, or a star, by Hadley’s quadrant. How far that promise was fulfilled, and the practicability of the instructions, are points worth consideration, as the book took a prominent place for some years. The errors which he said were inseparable from the dead-reckoning “even in the hands of the ablest and most skilful navigators,” amounting at times to 15 degrees, appear to be overestimated. On the other hand, the equations to determine the moon’s position at time of observation from Mayer’s tables, would, he believed, always determine the longitude within a degree, and generally to half a degree, if applied to careful observations. He recommends the two altitudes and distance being taken simultaneously when practicable. The probable error of observation in a meridian altitude he estimated at one or two minutes, and in a lunar distance at two minutes. He then gave clear rules for finding the moon’s position and distance by ten equations, too laborious for seamen to undertake. Admitting the requisite calculations for finding the moon’s place to be difficult, he desired to see the moon’s longitude and latitude computed for every twelve hours, and hence her distance from the sun and from a proper star on each side of her carefully calculated for every six hours, and published beforehand.
In 1765 Maskelyne became astronomer-royal, and was able to give effect to his own suggestion by organizing the publication of the Nautical Almanac. The same act of 1765 which gave Harrison his first £10,000 gave the commissioners authority and funds for this undertaking. Mayer’s tables, with his MS. improvements up to his death in 1762, were bought from his widow for £3000; £300 was granted to the mathematician L. Euler, on whose theory of the moon Mayer’s later tables were formed; and the first Nautical Almanac, that for 1767, was published in the previous year, at the cost and under the authority of the commissioners of longitude. In 1696 the French nautical almanac for the following year appeared, an improvement on what had been before issued by private persons, but it did not attempt to give lunar distances! In the English Nautical Almanac for 1767 we find everything necessary to render it worthy of confidence, and to satisfy every requirement at sea. The great achievement was that of giving the distance from the moon's centre to the sun, when suitable, and to about seven fixed stars, every three hours. The mariner has only to find the apparent time at ship, and clear his ow11 measured lunar distance from the effects of parallax and refraction (for which at the end of the book are given the methods of Lyons and Dunthorne), and then by simple proportions, or proportional logarithms, find the time at Greenwich. The calculations respecting the sun and moon were made from Mayer's last manuscript tables under the inspection of Maskelyne, and were so continued till 1804.2 The calculations respecting the planets are from Halley's tables, and those of Jupiter's satellites from tables made by Wargentin and published by Lalande in 1759 (except those for the fourth satellite). The original Nautical Almanac contained all the principal points of information which the searnan required, but the great value of such an authentic publication to the whole astronomical world led soon to a considerable increase to its contents. As much of this was unnecessary for the ordinary requirements of navigation, since 1903 it has been issued in two forms, the larger for observatory purposes, the Smaller for the class for whom it was originally intended.
Various useful rules and tables were appended to early volumes of the Almanac. Thus that for 1771 contains a method and table for determining the latitude by two altitudes and the elapsed time (first published by Cornelius Downes of Amsterdam in 1740). At the end of the Almanac for 1772 Maskelyne and Whichell gave three special tables for clearing the lunar distance; still their rule is neither short nor easily remembered. An improvement of Dunthorne's solution is also given. In the edition for 1773 a new table for equations of equal altitude was given by W. Wales. In those for 1797 and 1800 tables were added by John Brinkley for rendering the calculations for double altitudes easier.
The plan of the Nautical Almanac was soon imitated by other nations. In France the Académie Royale de Marine had all the lunar distances translated from the British Nautical Almanac for 1773 and following years, retaining Greenwich time for the three-hourly distances. The tables were considered excellent, and national pride was satisfied by their having been formed on the plan proposed by Lacaille. They did not imitate the mode given for clearing the lunar distance, considering their own better.
Though the Spaniards were leaders in the art of navigation during the 16th and 17th centuries, it was not till November 4, 1791, that their first nautical almanac was printed at Madrid, having been previously calculated at Cadiz for the year 1792. They acknowledge borrowing from the English and French. The excellent Berlin Aslronomisches Jahrbuch began to appear in 1776, the American Ephemeris in 1849. These two ephemerides and the French Cannaissance des temps are independent and valuable works.
A book of Tables Requisitc to be Used with the Nautical Effhemeris was published by Maskelyne at the same time as the first lmanac, and ten thousand copies were quickly sold. A second edition, prepared by Wales, appeared in 1781, an octavo of 237 pages, in the preface of which it is stated that it contains everything necessary for computing the latitude and longitude by observation. There are in all twenty-three tables, the traverse table and table of meridional arts alone being deficient as compared with modern works of the liind; dead-reckoning Maskelyne did not touch. He gave practical methods for working several problems; that for computing the lunar The French nautical almanac or Connaissance des temps appeared under letters patent from the king, dated 24th March 1679seventeen years before the first issue. The following is a literal translation of its advertisement: “ This little book is a collection of holy days and festivals in each month. The rising and setting of the rnoon when it is visible, and of the sun every day. The aspects of the planets as with respect to each other, the moon and the fixed stars. he lunations and eclipses. The difference of longitude between the meridian of Paris and the principal towns in France. The time of the sun's entrance into the twelve si ns of the zodiac. The true place of the planets every fifth day, and oithe moon every day of the year, in longitude and latitude. The moon's meridian assage, for finding the time of high water, ' as well as for the use of dials by moonlight A table of refraction. The equation of~ time [this table is strangely arranged, as though the clock were to be reset on the first of every month, and the explanation speaks of the ' premier mobile ']. The time of twilight at Paris. The sun's right ascension to hours and minutes. The sun's declination at noon each day to seconds. The whole accompanied by necessary instructions."
2 Mayer's tables were printed at London under Maskelyne's superintendence in 1770.
especially is an improvement on those by Lyons and Dunthorne, and a rule given for clearing the distance, called Dunthorne's improved method, is remarkably short. Maskelyne's rule for finding the latitudes by two altitudes and the elapsed time is also good. The third edition of the Tables was issued in 1802. The publication of the Requisite Tables met a great want, and the existence of such accurate and conveniently-arranged mathematical tables for the special purposes of nautical calculations led to the more general use of many refinements which had been previously neglected. They formed the original of many subsequent and greatly extended collections, of which those by J. W. Norie are the more generally used in modern times in the mercantile marine, and the very accurate and comprehensive tables by James Inman (originally published in 1823) are constantly used in the British navy.
Until the middle of the 17th century mariners generally employed small collections of Dutch charts, known as “ waggoners" from Waghenair, the name of a celebrated Dutch hydrographer in 1584. In 1671 appeared the English Pilot by John Sellers, who is styled the “ Hydrographer Royal.” It forms a collection of rude sketches of the coasts of England, the North Sea, France and Spain, with safiing directions, and on its appearance the importation of Dutch charts was prohibited. Private enterprise, for many years after that, supplied both the British navy and the British mercantile marine with constantly improving charts, especially latterly, under the powerful patronage of the East India Company, whose hydrographer (Alexander Dalrymple), in 1795, was selected as the first hydrographer of the Admiralty. This post has since been occupied by a succession of distinguished naval officers under whom have grown up a large school of able nautical surveyors, the results of whose labours are now published in the well-known Admiralty charts. Prior to the issue of charts by the Admiralty, the instructions to masters of vessels in the British navy enjoined them to “ provide such charts and instruments as they considered necessary for the safe navigation of the ship, ” while on the completion of a voyage of discovery it was customary for the results to be published for the Admiralty by private firms.
The establishment of the Admiralty Hydrographic Office in 179 5 marked a great step in the advancement of the art of navigation. On the 12th of August of that year an order in council placed all such nautical documents as were then in the possession of the Admiralty in charge of Dalrymple, whose catalogue, compiled for the use of the East India Company in 1 786, contained 347 charts between England, the Cape, India and China; thus the germ of the present hydro graphic department was established. The expense was then limited to £6 50 a year. The first official catalogue of Admiralty charts was issued in 1830, the total number being then 962.
After the close of the long devastating war in 1815 both trade and science revived, and several governments besides that of Great Britain saw the necessity of surveying the coasts in various parts of the globe; the greater portion of the work fell to the English hydro graphical department, which took under its charge nearly every place where the inhabitants were not able to do it for themselves. Since that time its career of usefulness has steadily developed, and it not merely undertakes the constant improvement of the charts of the whole world, but periodically issues for the use of the seafaring community a vast amount of most accurate and practical nautical information on the various closely allied subjects of navigation, tides, compass adjustment and ocean meteorology.
A knowledge of the times and heights of high and low water and the directions of the tidal streams due to those phenomena are in many parts of the world (and especially round our own coasts) of vital importance to navigation. The theory of the tides was first laid down by Newton and Laplace, and in Phil. Trans., 1683. there is an account of Flamsteed's tide table for London Bridge, which gave the times of each high tide on every day in the year. For a long subsequent period empirical tide tables for a few places in England were published by private individuals, but in 1832 the researches of Dr Whewell and Sir J. W. Lubbock enabled official t1de tables to be issued by the Admiralty. These have steadily advanced in detail and accuracy, being now in many cases based on continuous tidal observations for a whole lunar period of 18% years, and represent the practical epitome of our knowledge of the tides and tidal currents of the whole world. The formulae and tables on which these predictions are based are given in the introduction to each annual volume (see TIDE).
MODERN NAVIGATION
Having thus sketched the progress of the art of navigation from an early period to the present time, we will now describe the modern methods by which it is brought into practical use referring our readers for more technical information to the professional text-books enumerated at the end of this article. The great development in both size and speed of modern ships enormously increases the responsibilities of those who command and navigate them, and has led to a careful examination of the existing modes of determining a ship’s position at all times by day or night, both when in sight of land and on the open ocean. An examination of the present text-books on the subject of navigation shows how problems and methods which were formerly considered chiefly as theoretical exercises have now, from the altered conditions of the navigation of very fast ships, become methods of frequent practice, while corresponding improvements have been made in the instruments, such as compasses, charts and chronometers, by the aid of which more satisfactory results are now attained. Much has also been done to advance the study of this and its numerous allied subjects by the development of the Royal Naval College at Greenwich and the United Service Institution; also by the establishment of ship masters' societies (of which the well-known society in London is typical), where during the year valuable papers are read and useful discussions take place among those actually carrying out the practice of navigation.
In planning out in advance a long ocean voyage the experienced navigator would first, by laying down the track from port to port on a great circle chart, ascertain the shortest route between them, remembering that the greatest saving in distance over other routes is when the ports are far apart in longitude and both in high latitudes of the same name. On examining such a track in conjunction with the wind and current charts it will be seen what modifications the intervention of land, unfavourable currents or winds, ice or unduly high latitude render necessary, and such modified route would be finally adopted subject to possible change as the voyage progressed. The judgment formed on the best route to follow would also be largely influenced by the remarks in the volumes of Sailing directions or “Pilots” relating to the region about to be traversed, while among the many excellent modern publications of the Hydrographic Office of the Admiralty perhaps the Ocean Passage Book is one of the most generally useful, since, when used in combination with the admirable charts of suggested full-powered and auxiliary tracks, it very greatly assists all navigators in planning out a successful voyage. Finally the intended route would be transferred from the great circle chart to one on Mercator’s projection, which is the more convenient for purposes of navigation since in constructing the former for the sake of simplicity a projection of the coast’s surface is adopted on which great circles are correctly shown as straight lines (gnomonic), while for practical purposes in navigation such a representation on which a ship’s track when steering a continuous course (technically termed a rhumb line) is truly shown as a straight line (Mercator) is the most convenient, although in high latitudes giving a very distorted representation of the surface depicted. It is well to remember that on great circle charts rhumb lines become curves and great circles straight lines, and, vice versa, on Mercator charts, the rhumb line on each projection being that nearer to the equator, all meridians and the equator on both projections are shown as straight lines.
Ships rarely steer on great circles, which would generally theoretically involve continually altering course, but a series of chords of such circles are described of lengths such as involve a practical change of course of one or two degrees on the completion of each.
Great circle charts are very useful for drawing what is known as a composite track where if the great circle route would lead into too high a latitude the shortest route to and from the highest desirable parallel is readily laid down, the intervening track being pursued on that parallel.
A method of drawing approximate great circles directly on Mercator charts was proposed by Airy in 1858, and is sometimes very useful. The excellent idea, originally suggested by M. F. Maury, of establishing steam “lanes” in localities where there is much ocean traffic, so as to minimize the risks of collision between outward and homeward bound ships, has been successfully carried out in the North Atlantic. The leading transatlantic steamship companies now agree to follow great circle routes from the Irish coast to points on the Banks of Newfoundland, which vary somewhat in position with the season of the year, but are published in advance. These “lanes” being avoided by sailing vessels, risks of collision are materially lessened.
Having thus planned the most desirable general track to pursue, three methods are employed to ascertain the position of the ship at any time during such voyage: these are (1) projecting the track on charts; (2) simple trigonometrical calculations where the data are the course steered and distance run; and (3) astronomical observations, which form an entirely independent method.
Of these the first is the least trustworthy, owing to the usual difficulties attending accurate graphic methods and the small scales on which ocean charts are necessarily drawn. When near the land the larger scale coast charts are used, and in the approaches to harbours still larger scale plans give increasing accuracy to this record of a ship’s position. Index charts of all parts of the world are provided, by referring to which the navigator ascertains which chart or plan to employ, always preferably using that on the largest scale.
On leaving harbour, and while near the coast, the position is not found by calculation but by frequently observing (when a variety of objects is in sight) (1) simultaneous sextant angles between suitably situated objects subsequently laid down on the chart by a station pointer; (2) simultaneous compass bearings of two or more objects (technically known as cross bearings); or (3) a combination of both methods by employing one bearing and one angle. All such methods are capable of considerable accuracy if the observations are made simultaneously. Should only a small number of objects, or sometimes only one, be visible (as frequently occurs at night) other and rougher methods are practised, depending upon the change of bearing of an object while a certain distance in a certain direction is traversed by the ship, such knowledge being based in many cases on an estimate of the action of the tide. When a ship is steaming at the rate of zo knots the navigator remembers that a mile is passed over in three minutes, and that if in sight of land and fixing positions by objects on shore, it is essential to adopt some rapid method; otherwise when laid down on the chart the position shows where the ship was, and not where she is. This difficulty has led to the more general use of methods of obtaining positions by angles instead of bearings, and laying them down on the chart by the aid of the station pointer. Many advantages accrue from this, as the observer is not restricted in position on board, as is the case when using the compass, and especially if a double sextant (having two index glasses and one horizon glass) is employed two angles can be measured simultaneously, the result on the chart being very rapidly arrived at. An ingenious combination of sextant and station pointer in one has been proposed, and most simply carried out by attaching vertical sights to the legs of a station pointer, which is put on a suitable horizontal stand, and the legs moved until the sights are in line with the objects observed. To assist the navigator in the choice of suitable objects between which to measure the angles, a very useful pamphlet is issued by the Admiralty, from the diagrams in which it can be seen at a glance which combination of objects in sight gives the most favourable result, always remembering as a broad principle that nearer objects are more suitable than distant ones, and that the accuracy of position determined depends on the relative distances of the objects as well as on the magnitude of the angles between them. In these circumstances which render these rougher methods those only available, and especially in hazy weather in many known localities (such as the English Channel), a continuous line of deep sea soundings at fairly even distances apart affords an additional verification of position, remembering that only an occasional sounding might prove very misleading. The chronicle of progress in the art of navigation would be very incomplete without reference to the extended use of Lord Kelvin’s sounding machines, either in the original form, where the increased pressure at different depths is recorded by discoloration of chemical tubes, or in the later form known as the “depth recorder,” where similar results are obtained by the automatic record of the position of a piston forced upwards in a tube by this increased pressure. Very satisfactory results can be obtained at speeds of 15 or 16 knots, enabling that great safeguard of navigation in many places, viz. a continuous line of soundings, to be accurately and rapidly obtained. In connexion with this should be mentioned a most ingenious invention known as the “submarine sentry,” which on being set for any desired depth and towed overboard remains at that depth whatever the speed of the ship may be. On striking bottom it at once floats to the surface and rings a warning bell. Such an instrument is of obvious value in ships where, owing to the small number of available men, it is difficult to maintain a continuous line of soundings. To avoid an unnecessarily wide détour in rounding points and shoals, extensive use is now made of both horizontal and vertical danger angles; the former is the angle on the arc of a horizontal circle passing through a point at the required distance from the danger, and through two previously selected, easily recognized, fixed objects. Should circumstances enable the selection to be made of an angle of about 9o°, the ship by continually measuring the angle may be steered on the arc of such a circle with great precision, and may even be safely taken through a channel between two dangers. The vertical danger angle enables similar results to be attained by measuring the vertical angle subtended by a known height; but except where the selected object is one whose height is well determined, such as a lighthouse, this method is not so trustworthy as the former.
Before losing sight of land the latitude and longitude of the last well-determined position found by the methods referred to is taken from the coast chart, transferred to the ocean or small scale chart, and considered to be the “departure” or starting-point of the ocean voyage, and from that point the course and distance run by the ship is laid down, being rectified on every occasion when the position is more accurately determined by astronomical means. To obviate the inevitable inaccuracies attending this graphic method and as a corroboration of the ship’s position, the changes of latitude and longitude involved in each alteration of course are daily calculated by plane trigonometry, such calculations being materially abbreviated by the use of the Traverse Table, which is a tabulated expression of the solutions of right-angled plane triangles.
The foregoing modes of keeping account of a ship’s position are technically known as “dead reckoning.” The general introduction of compasses with short needles and slow periods of vibration has done very much towards improving the accuracy with which a ship’s “dead reckoning” is kept. The original model of these was that patented by Lord Kelvin in 1876, and since adopted in the British navy as the standard. In this instrument we have a compass specially designed to enable the principles of compensation or correction proposed by Sir G. B. Airy in 1837 to be accurately carried out, while its slow period of swing renders it in all circumstances extremely steady.
The record of distance run is always obtained from the patent log, usually in the form of the Cherub or Taffrail log introduced in 1878. The common or hand log has ceased to be regarded as anything but the very roughest of guides, and the patent log in its original form, in which it recorded the revolutions of a small screw towed by the ship, does not give satisfactory results at great speeds, nor can anything more favourable be said of those forms where pressure on known areas is employed. The revolutions of the engines, with due allowance made for the condition of the ship’s bottom, afford now perhaps the best means of estimating speed (see Log).
Astronomical observations afford the most accurate means of ascertaining positions at sea, other methods (dead reckoning) being only relied upon when the weather does not admit of the practice of these, though by utilizing twilight and night observations of moon, stars and planets, the navigator in most parts of the world need seldom proceed far without the means of astronomically rectifying his position either in latitude, longitude or both at the same time.
The practical problems involved are precisely those employed at astronomical Observatories, but it is not possible to attain similar accuracy of results, for though the sextant (the instrument always employed at sea in making such observations) is capable of marvellous accuracy, yet, as practically all such observations depend directly upon altitudes measured above the sea horizon, the uncertainty and variability of the true position of this, due to the changing effects of refraction, much affect observations made' at any one time. This error in practice is greatly reduced by methods of combining several observations made at different times and using their mean or average result. A notable feature of the progress of the art of modern navigation is the greatly increased practice of star navigation, and many of the supposed difficulties of night observations are found to be removed by experience. Determinations of positions at sea by twilight observations, when the brighter stars become visible while the horizon is still well denned, are probably the most accurate means we possess; and the careful navigator, by combining for latitude stars passing north and south of the zenith, and for longitude those near the prime vertical both east and west, can generally depend upon a good result, especially if suitable stars can be found for each pair at about the same altitudes. For these purposes the armillary sphere is extremely useful: this is a small celestial globe on which are depicted the principal stars visible to the naked eye. On elevating the pole to the approximate latitude of the observer, and turning the sphere until the sidereal time is under the fixed meridian, a correct representation of the heavens at the time of observation is obtained; the stars are then easily identified by their bearings and altitudes. This valuable instrument is not merely useful when at twilight, only a few of the brighter stars being visible, the constellations to which they belong are difficult of recognition, but it enables arrangement to be made in advance for such observations as are desired to be taken during the night. By marking in pencil on the globe the positions of the planets in right ascension and declination, the same sphere is also available for their identification. The heavenly bodies commonly observed at sea are: The Sun, Moon, Venus, Mars, Jupiter, Saturn, the Pole star, and the larger (or first magnitude) fixed stars, the positions of all of which in the heavens are given in the Nautical Almanac for fixed epochs at Greenwich, with the requisite data for computing their positions at all other times in all other places.
The chief astronomical observations made at sea are those for ascertaining (1) latitude, (2) time and thence longitude, (3) error of compass, and (4) latitude and longitude simultaneously.
To ascertain latitude by itself altitudes of heavenly bodies are measured above the horizon when they are on or near the meridian and therefore exactly or nearly north or south of the observer; in the case of the sun, of course, this means at or near noon, and in the case of other bodies such local times are previously accurately ascertained by a simple calculation made from the Nautical Almanac or more roughly found from an armillary sphere. The principle involved is the simple one that by subtracting the observed altitude when on the meridian from 90° the distance of the zenith or point overhead north or south of the heavenly body is found; then by combining with this the distance, obtained from the Nautical Almanac, of the body considered north or south of the celestial equator at the same instant, it is found how far the zenith is north or south of the celestial equator, and this is exactly the same as the latitude of the observer since the celestial equator is merely the imaginary extension of that of the earth. Such observations are not necessarily restricted to that which can be taken at the instant when the body observed is on the meridian (meridian altitude); equally accurate and multiplied observations can be made on either or both sides of the meridian if the body is somewhat near it (ex-meridian and circum-meridian altitudes), and a simple calculation or reference to a specially constructed table or graphic curve gives the required result.
Errors arising from uncertainty as to the true position of the horizon are with twilight and night observations largely counteracted by taking the means of results obtained from observations made of heavenly bodies crossing the meridian both north and south of the observer, taken as nearly at the same time as convenient. In northern latitudes the pole star is so near to the pole that observations of it can be taken at any time when it is visible, and from a convenient table given in the Nautical Almanac the altitude of the pole itself (which equals the latitude) is readily obtained.
Longitude at sea is in modern navigation always found by comparing local or ship mean time with Greenwich mean time, the latter being accurately known from the chronometers and the former from astronomical observations of suitably placed heavenly bodies. It may be assumed in all well found modern ships that on applying the known errors and accumulated rates to the times shown by the chronometers the Greenwich time at any instant is practically accurately known, and as the distance east or west of any place is merely the difference between the two local times at any instant expressed in degrees, so also is the distance east or west of Greenwich (longitude) the difference between time at place and Greenwich time at any one instant. The connexion between time and degrees depends upon the complete rotation of the earth in twenty-four hours, causing meridians 15° apart to pass under the same fixed point in the heavens at intervals of one hour, those east of Greenwich passing earlier and those west later, resulting in local time being in advance of Greenwich time in east longitude and vice versa in west longitude.
The errors and rates of gaining or losing of the chronometer referred to are known from observations made on shore prior to the beginning of the voyage with a sextant and artificial horizon, and these observations are capable of almost as great accuracy as those taken at fixed astronomical observatories. As this knowledge is absolutely essential every opportunity is taken at each principal port visited of either repeating such observations or obtaining the information from time balls dropped from observatories on shore at the Greenwich times indicated in the Time-ball pamphlet. Local or ship time can only be found with fair accuracy from calculations based on altitudes of heavenly bodies, when they are nearly east or west of the observer or technically on the prime vertical. Such times can be approximately seen from the azimuth diagrams or from tables of true bearings of heavenly bodies, and the error involved by uncertainty as to the position of the horizon can be greatly obviated in twilight or at night by taking the mean of results arising from nearly simultaneous observations of bodies bearing both east and west. In the usual case of determining time by observations of the sun the results arising from morning observations are compared with those similarly obtained in the afternoon. It will of course be remarked that should any unhallowed-for error in the chronometer exist it will affect the resulting longitude by its full amount.
In considering the foregoing methods of astronomically fixing a ship’s position we notice that always when the two elements of latitude and longitude are determined at different times, and generally, as we shall presently see, when they are determined together (though usually for a shorter time) the navigator has to depend for some time on the accuracy of the course steered and estimated distance run; also when cloudy weather prevails he has to depend entirely on those elements for a knowledge of the ship’s position. The frequent astronomical observation of the error of the compass is therefore a most important and fortunately simple duty. In practice the error is found by a comparison between the compass bearing of a heavenly body and its true bearing, obtained either by calculation, or more generally from a graphic diagram (Weir’s azimuth diagram) or tables from which at practically any time when above the horizon the true bearings of the principal heavenly bodies are taken by inspection. These important observations are most accurately made when the body observed is bearing nearly east or west true, if not too high, but if clouds prevent observations at such times, fairly good results can be obtained by observing the compass bearing when the object is on the meridian (if not too high) and therefore lying north or south true.
The causes of the changing errors of a compass in an iron ship are described elsewhere (see Compass), but by making comparisons as above the navigator can at once ascertain what is termed the “total” error, and if he takes from that the portion of error due to the earth, or what is termed variation (known from a chart of such elements), the remaining error is that caused by the iron of the ship, technically known as deviation. The latter method of procedure has the great advantage of enabling the navigator to ascertain during a voyage whatever magnetic changes in the ship are taking place other than those he would expect to occur on change of position. The total error is that applied to compass courses.
Deviations greater than a few degrees are not merely inconvenient but in modern compasses produce unsteadiness or oscillation of the compass card, so that, especially in new ships, the skilful navigator reduces such errors by adjusting the compensating magnets when favourable occasions offer. Recognizing the great value of a sound knowledge of compass adjustment, the British Board of Trade have included this among the compulsory subjects of examination for the rank of master, thus following the example of the navy, where all navigating officers have to attend a practical course of study on the subject.
The practical problem of finding both latitude and longitude at the same time is the most important of all in modern navigation, and is rapidly superseding other modes of ascertaining a ship’s position. The principle involved depends upon the fact that every heavenly body is at each particular instant of time directly overhead or in the zenith of some place on the earth. Thus, if we take the sun as an instance, it is noon at all places on the meridian of 60° W. when it is exactly 4 p.m. at Greenwich, and at the one spot on that meridian where the observer is as far north or south of the terrestrial equator as the sun is north or south of the celestial equator (declination) it will not only be noon but the sun will be immediately overhead and will have an altitude of 90°. This, therefore, at any instant defines the position where the sun is vertical; its latitude must equal the sun’s declination and its longitude in time equal the time since noon at Greenwich. Now at distance of 60 m. in every direction on the surface of the earth from the point thus defined the sun will have an altitude of 89° and in all directions at a distance of 1200 m. its altitude will be 70° (=90°−20°), so that on a globe, by marking the position where at a certain instant the sun is vertical and taking that as a centre, a series of concentric circles may be drawn, on all points of each of which the sun’s altitude will be the same. When, therefore, at sea we measure with a sextant at any time the altitude of the sun (say 60° 10′) we at once know we are somewhere on the arc of a circle having for its centre the spot where the sun is vertical at that instant, and for radius a distance equal to 1790′ (=90°−60° 10′). Such information, combined with the best and most recent knowledge we have of the ship’s latitude at the time, will of itself afford valuable information as to the position, but by making two such observations, separated by a sufficiently long interval for the position having the sun vertical to have moved considerably (owing to the rotation of the earth), we are able to consider with certainty that we must be at one or other of the widely separated intersections of two such circles, the movement of the ship in the interval between the two observations being duly allowed for. The dead reckoning affords information as to which of these intersections is the true position.
Now even on a large globe it would be practically impossible to obtain very accurate results from this problem by drawing such circles, but on a large scale chart (or ordinary squared paper) much greater accuracy is obtainable. The method commonly used on a Mercator chart involves two suppositions: (1) that the concentric circles we have referred to will be correctly represented as circles on the chart, and (2) that these are of such diameters, that a portion of say 100 m. of arc may be considered to be a straight line coincident with the tangent to the circle and therefore at right angles to the direction of the sun. Except in high latitudes (above 60°) Mercator’s projection fulfils the first condition sufficiently well for practical purposes, and, except when the altitude is greater than 70°, the second condition is also approximately true since the radii of such circles will exceed 1200 m.
| An image should appear at this position in the text. If you are able to provide it, see Wikisource:Image guidelines and Help:Adding images for guidance. |
- Fig. 7.
Premising these conditions, suppose that on a certain day at 9 a.m. when the ship’s approximate position, known from previous observations and laid down on the chart, is supposed to be at A (fig. 7), an observation of the sun is made from which the longitude is calculated, the result being that on the supposition that the latitude of A is correct, the ship’s position is probably at B. Now by drawing a straight line ab through B at right angles to the true bearing of the sun at the time of observation (which is most readily known from the azimuth tables) we are obviously right in assuming the ship’s position to be somewhere on that line if we consider it as approximately an arc of a large circle having the place where the sun is then vertical as a centre, the direction of such place being indicated by an arrow.
If our supposed latitude be right the position will be at B, but if not correct it must still be on the line ab, and if near land or any danger the direction of this line, even if no subsequent observation be available, will often give most valuable information. If, while waiting for the sun to change its bearing, the ship runs from B to C, a line cd drawn through C parallel to ab will represent an arc on which the position lies when she is probably at C, which at this instant (10.30 a.m.) is the most probable position of the ship.
If another observation of the sun for longitude is now made and the resulting position is D (lying of course in the same latitude as C), on drawing through D a line ef at right angles to the bearing of the sun (indicated by an arrow) we are right in assuming the position to be somewhere on such an arc as is represented by this line.
Hence E, the intersection of the two arcs on which the position lies at the same instant, must be the true place when the last observation was taken at the supposed position D, the discrepancies being entirely due to the original unknown error in the assumed latitude of A, for had that been accurate the position on the original line ab would have been such that on laying off the course and distance from that position C would have coincided with E.
Errors in the assumed latitude of as much in many cases as 30 m. will often be found to produce no practical difference in the resultant position, but of course the accuracy of the longitude found is entirely dependent upon the chronometer, and in such cases as arise when the intersecting arcs make a small angle with each other great accuracy is required in the course and distance run between the times of observation.
This method of finding both latitude and longitude at the same time is commonly known as “Sumner's” method from the publicity given to it in 1847 by the publication of an excellent pamphlet on the subject by a master of that name in the American mercantile marine, although in a modified form it was practised at a much earlier date in the British navy under the name of “cross bearings of the sun.” Prior to the publication of azimuth tables in 1866 the calculation was more lengthy and troublesome, the work being practically doubled.
We have taken an illustration from observations of the sun, but the method is obviously applicable to all heavenly bodies provided they are so situated that the arcs drawn will intersect at a good angle; this in twilight or at night-time is readily done by selecting two heavenly bodies whose bearings differ considerably, and in such cases the small complication of allowing for the run of the shi is often obviated by making the observations simultaneously. The armillary sphere or star globe is useful in selecting objects suitably situated.
The principle of Sumner's method has of recent ears received a very important and valuable development under the name of the “new navigation.” In this method, originally proposed by Marc St Hilaire, a comparison is made between the altitude of a heavenly body as actually observed and that calculated from the supposed position of the ship. For instance, the position of an observer at the instant of observing a (true) altitude of the sun of 40° 10′ must be somewhere on a portion of the circumference of a circle (usually of such size that the portion considered may be represented on a chart by a straight line) having its centre in latitude equal to the sun's declination, and in longitude equal to the Greenwich apparent time at the instant, the radius of such a circle being equal to the sun's zenith distance of 49° 50′. If at the same time the true altitude of the sun is from the estimated position of the ship calculated to be 40° 5′, it is evident that the greater observed altitude must be owing to the ship being nearer to the centre of the circle than was supposed, and a line of position drawn through the estimated position at right angles to the bearing of the sun must be transferred parallel to itself through a distance of 5′ towards the direction of the sun's bearing. The second line of position, obtained when the sun's bearing has altered some 25°, is dealt with in a similar way, and the intersection of' the two lines so obtained gives the position of the ship at the time of second observation. This mode of procedure enables all observations, whether near or far from the meridian, to be similarly dealt with; in all cases the altitude the heavenly body should have is computed and compared with what it actually has. The practice of problems such as the foregoing is greatly facilitated by the extended means of finding at any moment the azimuth or true bearing of a heavenly body. When the azimuth was only required for the determination of compass error, the valuable tables from which the computed results could be obtained by inspection were limited to those cases of most practical importance, but from the ingenious and simple graphical form known as Weir's azimuth diagram azimuths of all heavenly bodies, whose declinations extend from 60° N. to 60° S., can be obtained during the whole time they are above the horizon, thus greatly facilitating the laying down lines of position.
A careful record of everything pertaining to the navigation of the ship, with the results of all observations and calculated positions, is kept in the ship's log, an official book of great importance, a rough original of which is kept on deck with entries made in it of all such events at the time of their occurrence. A copy of the headings of a page of this as transferred into the official log is here given:
| Hour. | Knots | Tenths of Knots. |
Course. | Wind. | Weather. | Deviation. | Barometer. | Thermometer | Temperature of Sea. |
Remarks. | |
| Direction. | Force. | ||||||||||
The course entered here is that which would be indicated by the “standard” compass, of the ship (placed in the most favourable magnetic position on board); that actually steered by is the one most conveniently seen by the helmsman. Comparisons between the latter and the “standard” are frequently made, their indications generally varying somewhat owing to the difference of deviation in different positions on the ship. The compass card is usually graduated into points and degrees, but the course is always estimated in degrees. The speed is ascertained from the indication of the patent log, the hand log being generally only used as a rough check on this. Wind direction and force are the result of estimation; as the speed and course of the ship so greatly affect the apparent direction and velocity no practical anemometer for use on board ship exists. Wind force is estimated in terms of what is known as the “Beaufort” scale, based on the supposed amount of sail a vessel could carry at the time. The height 0 the mercurial barometer is carefully read at the end of each watch, as also is the thermometer; the more sensitive aneroid barometer is kept in a very accessible position and, more frequently referred to by the officer of the watch. When navigating in localities and during seasons at which circular storms or hurricanes may be expected (as known from the Barometer Manual) the barometer is anxiously and frequently watched, and at all times its indication is compared with that normally experienced in the locality traversed as shown on the barometer charts, due allowance being made in the tropics for the ordinary daily movement. All observations relating to ocean meteorology are of great service in the compilation and improvement of wind and current charts, and in many ships more extensive meteorological journals are voluntarily kept on forms supplied by the Meteorological Office. A knowledge of the temperature of the surface of the sea is often of great practical use in navigation as giving warning of change in direction of the surface ocean current, especially in localities where there exist near to each other warm and ccld currents setting in different directions, as, for instance, near the edge of the Gulf Stream. As an indication of the vicinity of ice such observations are usually much less trustworthy. On the completion of the calculations giving the ship's position at noon each day the results are tabulated in the ship's log on the following form:
| Course made good. | Distance. | Latitude. | Longitude. | Variation Allowed. | True Bearings and Distance. | |
| Current. | Made Good. | Through the water. | D.R. Obs. | D.R. Obs. | ||
The course and distance made good each day are calculated by trigonometry between the best determined positions at two successive noons, such positions in fine weather being always those determined astronomically, and the current being considered the difference in the positions at noon as determined astronomically and as calculated by dead reckoning since the previous noon; such differences, however, obviously include the errors of all kinds. The latitude and longitude found by dead reckoning are entered under that heading (D.R.). The astronomical positions of latitude and longitude (entered as “obs” or “by observation”) are very seldom both determined at noon, but are carried up or back to that instant by calculation from the intervening dead reckoning. The variation allowed is taken from the published variation chart, on which the latest results of such observations are embodied at intervals of about ten years with the annual changes (as far as known) in different localities, thus enabling the navigator to obtain its value at intermediate dates. Finally the course and distance are calculated from the position of the ship at noon to either the port of destination or some prominent position or danger near to which the vessel must pass. This is entered under the heading “true bearings and distance.”
Authorities.—The following list of some writers of navigation whose works have not been already mentioned may be found useful to refer to: Thomas Addison, Arithmetical Navigation (1625)—he was the first to apply logarithms; Antonio de Najera (Lisbon, 1628) follows Nuñez and Cespedes, but corrects the declination of sun and stars; Sir R. Dudley, L’arcano del mare (1630–1646, 2nd ed., Florence, 1661)—too ponderous for the use of seamen; Sir Jonas Moore (1681)—one of the best books of the period; William Jones (1702)—a useful compendium containing trigonometry applied to the various sailings, the use of the log, and tables of logarithms; Pierre Jean Bouguer, Traité complet de la navigation (folio, 1698)—good but too large; Manuel Pimental, L'Arte de navegar (Lisbon, 1712); Pierre Bouguer, jun., Nouveau traité de navigation (1753)—without tables, published at the request of the minister of marine, improved and shortened in 1769 under the superintendence of the astronomer Lacaille; Nathaniel Colson, The Mariner’s New Calendar (1735)—a good book; Seller, Practical Navigation—a book very popular in its time (there was an edition as late as 1739); Samuel Dunn published good star charts and tables of latitude and longitude (1737), and framed concise rules for many problems on navigation (published by the board of longitude); John H. Moore, The Practical Navigator and Seaman's New Daily Assistant (1772)—very popular, and generally used in the British navy—the 18th and 19th editions (1810,1814) were improved by J. Dessiou; W. Wilson (Edinburgh, 1773)—a treatise of good repute at the time; Samuel Dunn, New Epitome of Practical Navigation, or Guide to the Indian Seas (1777)—for the longitude he depends chiefly on a variation chart from observations by East Indiamen, and he still makes no mention of the Nautical Almanac or of parallel rulers; Samuel Dunn (probably a son of the last named, 1781) is the last writer who gives instructions for the use of the astrolabe: he also wrote on “lunars” (1783, 1793), a name which was generally adopted about this time, and published an excellent traverse table (1785), and Daily Uses of the Nautical Sciences, (1790); Horsburgh, Directory for East India Voyages (1805); A. Mackay, The Complete Navigator (about 179I); 2nd ed. 1810)—there is no instruction for finding longitude by the chronometer. Kelly, Spherical Trigonometry and Nautical Astronomy (1796, 4th ed., 1813)—clear and simple; N. Bowditch, Practical Navigator (1800)—passed through many editions and is now (in a revised form) the official text-book of the United States navy; J. W. Norie, Epitome of Navigation (1803, 21st ed. 1878)—still a favourite in the mercantile marine from its simplicity, and because navigation can be learned from it without a teacher; T. Kerigan, The Young Navigator's Guide to Nautical Astronomy (1821); Inman, Epitome of Navigation (1821)—with an excellent volume of tables, formerly largely used in the British navy, 9th ed. (1854); E. Riddle, Navigation and Nautical Astronomy (3rd ed. 1824, 9th ed., by Escott, 1871), still worthy of its high reputation; J. T. Towson, Tables for Reduction of Ex-meridian Attitudes (4th ed. 1854), very useful; H. Raper, Practice of Navigation (1840, 10th ed. 1870), an excellent book; H. Evers, Navigation and Great Circle Sailing (1850), other works on the same subject by Merrifield and Evers (1868) and Evers (1875); R. M. Inskip, Navigation and Nautical Astronomy (1865), a useful book, without tables; T. H. Sumner, A Method of finding a Ship’s Position by two Observations and Greenwich Time by Chronometer—this is set forth as a novelty, but was published by Captain R. Owen, R.N., early in the century, and practised by many officers; H. W. Jeans, Navigation and Nautical Astronomy (1858); Harbord, Glossary of Navigation (1863, enlarged ed. 1883), a very excellent book of reference; W. C. Bergen, Practice and Theory of Navigation (1872); Sir W. Thomson, Navigation, a Lecture (1876), well worth reading; Lecky, Wrinkles in Navigation (1880); Martin, Navigation and Nautical Astronomy, sanctioned for use in the British navy. (W. R. M.*)
- ↑ Halley’s observations were published posthumously in 1742 and in 1765 the commissioners of longitude paid his daughter £100 for MSS. supposed to be useful to navigation. As the moon passes the stars lying in her course through the heavens at the mean rate of 33″ in one minute of time, it is obvious that an error to that amount in measuring the distance from a star would produce an error of 15 m. in longitude. As the moon’s motion with regard to the sun is nearly one degree a day less, a similar error in the distance would produce still more effect.
- ↑ This total comprises the large sums awarded to Harrison and to the widow of Mayer, the cost of surveys and expeditions in various parts of the globe, large outlays on the Nautical Almanac and on subsidiary calculations and tables, rewards for new methods and solutions of problems, and many minor grants to watchmakers or for improvements in instruments. Thus Jesse Ramsden received in 1775 and later about £1600 for his improvements in graduation (q.v.), and E. Massey in 1804 got £200 for his log (see Log).
- ↑ The explanations and drawings are at the British Museum; and two of his watches, one of which was used by Captain Cook in the “Resolution,” are at Greenwich Observatory. In 1767 Harrison estimates that a watch could be made for £100, and ultimately for £70 or £80.