History (1)
In classical and medieval times bridges were constructed of timber or masonry, and later of brick or concrete. Then late in the 18th century wrought iron began to be used, at first in combination with timber or cast iron. Cast iron was about the same time used for arches, and some of the early railway bridges were built with cast iron girders. Cast iron is now only used for arched bridges of moderate span. Wrought iron was used on a large scale in the suspension road bridges of the early part of the 19th century. The great girder bridges over the Menai Strait and at Saltash near Plymouth, erected in the middle of the 19th century, were entirely of wrought iron, and subsequently wrought iron girder bridges were extensively used on railways. Since the introduction of mild steel of greater tenacity and toughness than wrought iron (i.e. from 1880 onwards) it has wholly superseded the latter except for girders of less than 100 ft. span. The latest change in the material of bridges has been the introduction of ferro-concrete, armoured concrete, or concrete strengthened with steel bars for arched bridges. The present article relates chiefly to metallic bridges. It is only since metal has been used that the great spans of 500 to 1800 ft. now accomplished have been made possible.
2. In a bridge there may be distinguished the superstructure and the substructure. In the former the main supporting member or members may be an arch ring or arched ribs, suspension chains or ropes, or a pair of girders, beams or trusses. The bridge flooring rests on the supporting members, and is of very various types according to the purpose of the bridge. There is also in large bridges wind-bracing to stiffen the structure against horizontal forces. The substructure consists of (a) the piers and end piers or abutments, the former sustaining a vertical load, and the latter having to resist, in addition, the oblique thrust of an arch, the pull of a suspension chain, or the thrust of an embankment; and (b) the foundations below the ground level, which are often difficult and costly parts of the structure, because the position of a bridge may be fixed by considerations which preclude the selection of a site naturally adapted for carrying a heavy structure.
3. Types of Bridges.—Bridges may be classed as arched bridges, in which the principal members are in compression; suspension bridges, in which the principal members are in tension; and girder bridges, in which half the components of the principal members are in compression and half in tension. But there are cases of bridges of mixed type. The choice of the type to be adopted depends on many and complex considerations:—(1) The cost, having regard to the materials available. For moderate spans brick, masonry or concrete can be used without excessive cost, but for longer spans steel is more economical, and for very long spans its use is imperative. (2) The importance of securing permanence and small cost of maintenance and repairs has to be considered. Masonry and concrete are more durable than metal, and metal than timber. (3) Aesthetic considerations sometimes have great weight, especially in towns. Masonry bridges are preferable in appearance to any others, and metal arch bridges are less objectionable than most forms of girder.
Most commonly the engineer has to attach great importance to the question of cost, and to design his structure to secure the greatest economy consistent with the provision of adequate strength. So long as bridge building was an empirical art, great waste of material was unavoidable. The development of the theory of structures has been largely directed to determining the arrangements of material which are most economical, especially in the superstructure. In the case of bridges of large span the cost and difficulty of erection are serious, and in such cases facility of erection becomes a governing consideration in the choice of the type to be adopted. In many cases the span is fixed by local conditions, such as the convenient sites for piers, or the requirements of waterway or navigation. But here also the question of economy must be taken into the reckoning. The cost of the superstructure increases very much as the span increases, but the greater the cost of the substructure, the larger the span which is economical. Broadly, the least costly arrangement is that in which the cost of the superstructure of a span is equal to that of a pier and foundation.
For masonry, brick or concrete the arch subjected throughout to compression is the most natural form. The arch ring can be treated as a blockwork structure composed of rigid voussoirs. The stability of such structures depends on the position of the line of pressure in relation to the extrados and intrados of the arch ring. Generally the line of pressure lies within the middle half of the depth of the arch ring. In finding the line of pressure some principle such as the principle of least action must be used in determining the reactions at the crown and springings, and some assumptions must be made of not certain validity. Hence to give a margin of safety to cover contingencies not calculable, an excess of material must be provided. By the introduction of hinges the position of the line of resistance can be fixed and the stress in the arch ring determined with less uncertainty. In some recent masonry arched bridges of spans up to 150 ft. built with hinges considerable economy has been obtained.
For an elastic arch of metal there is a more complete theory, but it is difficult of application, and there remains some uncertainty unless (as is now commonly done) hinges are introduced at the crown and springings.
In suspension bridges the principal members are in tension, and the introduction of iron link chains about the end of the 18th century, and later of wire ropes of still greater tenacity, permitted the construction of road bridges of this type with spans at that time impossible with any other system of construction. The suspension bridge dispenses with the compression member required in girders and with a good deal of the stiffening required in metal arches. On the other hand, suspension bridges require lofty towers and massive anchorages. The defect of the suspension bridge is its flexibility. It can be stiffened by girders and bracing and is then of mixed type, when it loses much of its advantage in economy. Nevertheless, the stiffened suspension bridge will probably be the type adopted in future for very great spans. A bridge on this system has been projected at New York of 3200 ft. span.
The immense extension of railways since 1830 has involved the construction of an enormous number of bridges, and most of these are girder bridges, in which about half the superstructure is in tension and half in compression. The use of wrought iron and later of mild steel has made the construction of such bridges very convenient and economical. So far as superstructure is concerned, more material must be used than for an arch or chain, for the girder is in a sense a combination of arch and chain. On the other hand, a girder imposes only a vertical load on its piers and abutments, and not a horizontal thrust, as in the case of an arch or suspension chain. It is also easier to erect.
A fundamental difference in girder bridges arises from the mode of support. In the simplest case the main girders are supported at the ends only, and if there are several spans they are discontinuous or independent. But a main girder may be supported at two or more points so as to be continuous over two or more spans. The continuity permits economy of weight. In a three-span bridge the theoretical advantage of continuity is about 49% for a dead load and 16% for a live load. The objection to continuity is that very small alterations of level of the supports due to settlement of the piers may very greatly alter the distribution of stress, and render the bridge unsafe. Hence many multiple-span bridges such as the Hawkesbury, Benares and Chittravatti bridges have been built with independent spans.
Fig. 1.—Trajan’s Bridge.
Lastly, some bridges are composed of cantilevers and suspended girders. The main girder is then virtually a continuous girder hinged at the points of contrary flexure, so that no ambiguity can arise as to the stresses.
Whatever type of bridge is adopted, the engineer has to ascertain the loads to be carried, and to proportion the parts so that the stresses due to the loads do not exceed limits found by experience to be safe. In many countries the limits of working stress in public and railway bridges are prescribed by law. The development of theory has advanced pari passu with the demand for bridges of greater strength and span and of more complex design, and there is now little uncertainty in calculating the stresses in any of the types of structure now adopted. In the modern metal bridge every member has a definite function and is subjected to a calculated straining action. Theory has been the guide in the development of bridge design, and its trustworthiness is completely recognized. The margin of uncertainty which must be met by empirical allowances on the side of safety has been steadily diminished.
Fig. 2.—Bridge of Alcantara.
The larger the bridge, the more important is economy of material, not only because the total expenditure is more serious, but because as the span increases the dead weight of the structure becomes a greater fraction of the whole load to be supported. In fact, as the span increases a point is reached at which the dead weight of the superstructure becomes so large that a limit is imposed to any further increase of span.
Fig. 3.—Ponte Salario.
History of Bridge Building
4. Roman Bridges.—The first bridge known to have been constructed at Rome over the Tiber was the timber Pons Sublicius, the bridge defended by Horatius. The Pons Milvius, now Ponte Molle, was reconstructed in stone by M. Aemilius Scaurus in 109 B.C., and some portions of the old bridge are believed to exist in the present structure. The arches vary from 51 to 79 ft. span. The Pons Fabricius (mod. Ponte dei Quattro Capi), of about 62 B.C., is practically intact; and the Pons Cestius, built probably in 46 B.C., retains much of the original masonry. The Pons Aelius, built by Hadrian A.D. 134 and repaired by Pope Nicholas II. and Clement IX., is now the bridge of St Angelo. It had eight arches, the greatest span being 62 ft.[1] Dio Cassius mentions a bridge, possibly 3000 to 4000 ft. in length, built by Trajan over the Danube in A.D. 104. Some piers are said still to exist. A bas-relief on the Trajan column shows this bridge with masonry piers and timber arches, but the representation is probably conventional (fig. 1). Trajan also constructed the bridge of Alcantara in Spain (fig. 2), of a total length of 670 ft., at 210 ft. above the stream. This had six arches and was built of stone blocks without cement. The bridge of Narses, built in the 6th century (fig. 3), carried the Via Salaria over the Anio. It was destroyed in 1867, during the approach of Garibaldi to Rome. It had a fortification such as became usual in later bridges for defence or for the enforcement of tolls. The great lines of aqueducts built by Roman engineers, and dating from 300 B.C. onwards, where they are carried above ground, are arched bridge structures of remarkable magnitude (see Aqueducts, § Roman). They are generally of brick and concrete.
Fig. 4.—First Span of Schaffhausen Bridge.
5. Medieval and other Early Bridges.—Bridges with stone piers and timber superstructures were no doubt constructed from Roman times onward, but they have perished. Fig. 4 shows a timber bridge erected by the brothers Grubenmann at Schaffhausen about the middle of the 18th century. It had spans of 172 and 193 ft., and may be taken as a representative type of bridges of this kind. The Wittingen bridge by the same engineers had a span of 390 ft., probably the longest timber span ever constructed. Of stone bridges in Great Britain, the earliest were the cyclopean bridges still existing on Dartmoor, consisting of stone piers bridged by stone slabs. The bridge over the East Dart near Tavistock had three piers, with slabs 15 ft. by 6 ft. (Smiles, Lives of the Engineers, ii. 43). It is reputed to have lasted for 2000 years.

Fig. 5.—Crowland Bridge.
The curious bridge at Crowland near Peterborough (fig. 5) which now spans roadways, the streams which formerly flowed under it having been diverted, is one of the earliest known stone bridges in England. It is referred to in a charter of the year 943. It was probably built by the abbots. The first bridges over the Thames at London were no doubt of timber. William of Malmesbury mentions the existence of a bridge in 994. J. Stow (Survey of the Cities of London and Westminster) describes the building of the first stone bridge commonly called Old London Bridge: “About the year 1176, the stone bridge was begun to be founded by Peter of Colechurch, near unto the bridge of timber, but more towards the west.” It carried timber houses (fig. 6) which were frequently burned down, yet the main structure existed till the beginning of the 19th century. The span of the arches ranged from 10 to 33 ft., and the total waterway was only 337 ft. The waterway of the present London Bridge is 690 ft., and the removal of the obstruction caused by the old bridge caused a lowering of the low-water level by 5 ft., and a considerable deepening of the river-bed. (See Smiles, Lives of the Engineers, “Rennie.”)

|
| From J. R Green’s A Short History of the English People, by permission of Macmillan & Co., Ltd. |
| Fig. 6.—Old London Bridge, A.D. 1600. From a Drawing in the Pepysian Library Magdalene College, Cambridge. |
The architects of the Renaissance showed great boldness in their designs. A granite arch built in 1377 over the Adda at Trezzo had a span at low water of 251 ft. This noble bridge was destroyed for military reasons by Carmagnola in 1416. The Rialto bridge at Venice, with a span of 91 ft., was built in 1588 by Antonio da Ponte. Fig. 7 shows the beautiful Ponte dellà Trinità erected at Florence in 1566 from the design of B. Ammanati.
6. Modern Bridges.—(a) Timber.—In England timber bridges of considerable span, either braced trusses or laminated arches (i.e. arches of planks bolted together), were built for some of the earlier railways, particularly the Great Western and the Manchester, Sheffield & Lincolnshire. They have mostly been replaced, decay having taken place at the joints. Timber bridges of large span were constructed in America between the end of the 18th and the middle of the 19th century. The Amoskeag bridge over the Merrimac at Manchester, N.H., U.S.A., built in 1792, had 6 spans of 92 ft. The Bellows Falls bridge over the Connecticut (built 1785–1792) had 2 spans of 184 ft. The singular Colossus bridge, built in 1812 over the Schuylkill, a kind of flat arched truss, had a span of 340 ft. Some of these timber bridges are said to have lasted ninety years with ordinary repairs, but they were road bridges not heavily loaded. From 1840, trusses, chiefly of timber but with wrought-iron tension-rods and cast-iron shoes, were adopted in America. The Howe truss of 1830 and the Pratt truss of 1844 are examples. The Howe truss had timber chords and a lattice of timber struts, with vertical iron ties. In the Pratt truss the struts were vertical and the ties inclined. Down to 1850 such bridges were generally limited to 150 ft. span. The timber was white pine. As railway loads increased and greater spans were demanded, the Howe truss was stiffened by timber arches on each side of each girder. Such a composite structure is, however, fundamentally defective, the distribution of loading to the two independent systems being indeterminate. Remarkably high timber piers were built. The Genesee viaduct, 800 ft. in length, built in 1851–1852 in 10 spans, had timber trestle piers 190 ft. in height. (See Mosse, “American Timber Bridges,” Proc. Inst. C.E. xxii. p. 305, and for more modern examples, cxlii. p. 409; and clv. p. 382; Cooper, “American Railroad Bridges,” Trans. Am. Soc. C.E. vol. xxi pp. 1-28.) These timber framed structures served as models for the earlier metal trusses which began to be used soon after 1850, and which, except in a few localities where iron is costly, have quite superseded them.
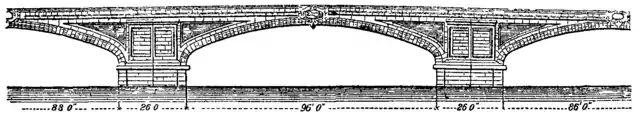
Fig. 7.—Ponte della Trinità, Florence.
7. (b) Masonry.—The present London Bridge, begun in 1824 and completed in 1831, is as fine an example of a masonry arch structure as can be found (figs. 8 and 9). The design was made by John Rennie the elder, and the acting engineer was his son, Sir John Rennie. The semi-elliptical shape of the arches the variation of span, the slight curvature of the roadway, and the simple yet bold architectural details, combine to make it a singularly beautiful bridge. The centre arch has a span of 152 ft., and rises 29 ft. 6 in above Trinity high-water mark; the arches on each side of the centre have a span of 140 ft. and the abutment arches 130 ft. The total length of the bridge is 1005 ft., its width from outside to outside 56 ft., and height above low water 60 ft. The two centre piers are 24 ft. thick, the exterior stones are granite, the interior, half Bramley Fall and half from Painshaw, Derbyshire. The voussoirs of the centre arch (all of granite) are 4 ft. 9 in. deep at the crown, and increase to not less than 9 ft. at the springing. The general depth at which the foundations are laid is about 29 ft. 6 in. below low water. The total cost was £1,458,311, but the contractor’s tender for the bridge alone was £425,081.
Fig. 8.—London New Bridge.
Since 1867 it had been recognized that London Bridge was inadequate to carry the traffic passing over it, and a scheme for widening it was adopted in 1900. This was carried out in 1902–1904, the footways being carried on granite corbels, on which are mounted cornices and open parapets. The width between parapets is now 65 ft., giving a roadway of 35 ft. and two footways of 15 ft. each. The architect was Andrew Murray and the engineer, G. E. W. Cruttwell. (Cole, Proc. Inst. C.E. clxi. p. 290.)
The largest masonry arch is the Adolphe bridge in Luxemburg, erected in 1900–1903. This has a span of 278 ft., 138 ft. rise above the river, and 102 ft. from foundation to crown. The thickness of the arch is 4 ft. 8 in. at the crown and 7 ft. 2 in. where it joins the spandrel masonry. The roadway is 52 ft. 6 in. wide. The bridge is not continuous in width, there are arch rings on each face, each 16.4 ft. wide with a space between of 19.7 ft. This space is filled with a flooring of reinforced concrete, resting on the two arches, and carrying the central roadway. By the method adopted the total masonry has been reduced one-third. One centering was used for the two arch rings, supported on dwarf walls which formed a slipway, along which it was moved after the first was built.

Fig. 9.—Half Elevation and Half Section of Arch of London Bridge.
Till near the end of the 19th century bridges of masonry or brickwork were so constructed that they had to be treated as rigid blockwork structures. The stability of such structures depends on the position of the line of pressure relatively to the intrados and extrados of the arch ring. Generally, so far as could be ascertained, the line of pressure lies within the middle half of the depth of the voussoirs. In finding the abutment reactions some principle such as the principle of least action must be used, and some assumptions of doubtful validity made. But if hinges are introduced at crown and springings, the calculation of the stresses in the arch ring becomes simple, as the line of pressures must pass through the hinges. Such hinges have been used not only for metal arches, but in a modified form for masonry and concrete arches. Three cases therefore arise: (a) The arch is rigid at crown and springings; (b) the arch is two-hinged (hinges at springings); (c) the arch is three-hinged (hinges at crown and springings). For an elementary account of the theory of arches, hinged or not, reference may be made to a paper by H. M. Martin (Proc. Inst. C. E. vol. xciii. p. 462); and for that of the elastic arch, to a paper by A. E. Young (Proc. Inst. C.E. vol. cxxxi. p. 323).
In Germany and America two- and three-hinged arches of masonry and concrete have been built, up to 150 ft. span, with much economy, and the calculations being simple, an engineer can venture to work closely to the dimensions required by theory. For hinges, Leibbrand, of Stuttgart, uses sheets of lead about 1 in. thick extending over the middle third of the depth of the voussoir joints, the rest of the joints being left open. As the lead is plastic this construction is virtually an articulation. If the pressure on the lead is uniformly varying, the centre of pressure must be within the middle third of the width of the lead; that is, it cannot deviate from the centre of the voussoir joint by more than one-eighteenth of its depth. In any case the position of the line of pressures is confined at the lead articulations within very narrow limits, and ambiguity as to the stresses is greatly diminished. The restricted area on which the pressure acts at the lead joints involves greater intensity of stress than has been usual in arched bridges. In the Württemberg hinged arches a limit of stress of 110 tons per sq. ft. was allowed, while in the unhinged arches at Cologne and Coblentz the limit was 50 to 60 tons per sq. ft. (Annales des Fonts et Chaussées, 1891). At Rechtenstein a bridge of two concrete arches has been constructed, span 7512 ft., with lead articulations: width of arch 11 ft.; depth of arch at crown and springing 2.1 and 2.96 ft. respectively. The stresses were calculated to be 15, 17 and 12 tons per sq. ft. at crown, joint of rupture, and springing respectively. At Cincinnati a concrete arch of 70 ft. span has been built, with a rise of 10 ft. The concrete is reinforced by eleven 9-in. steel-rolled joists, spaced 3 ft. apart and supported by a cross-channel joist at each springing. The arch is 15 in. thick at the crown and 4 ft. at the abutments. The concrete consisted of 1 cement, 2 sand and 3 to 4 broken stone. An important series of experiments on the strength of masonry, brick and concrete structures will be found in the Zeitschr. des österreichen Ing. und Arch. Vereines (1895).
The thermal coefficient of expansion of steel and concrete is nearly the same, otherwise changes of temperature would cause shearing stress at the junction of the two materials. If the two materials are disposed symmetrically, the amount of load carried by each would be in direct proportion to the coefficient of elasticity and inversely as the moment of inertia of the cross section. But it is usual in many cases to provide a sufficient section of steel to carry all the tension. For concrete the coefficient of elasticity E varies with the amount of stress and diminishes as the ratio of sand and stone to cement increases. Its value is generally taken at 1,500,000 to 3,000,000 ℔ per sq. in. For steel E = 28,000,000 to 30,000,000, or on the average about twelve times its value for concrete. The maximum compressive working stress on the concrete may be 500 ℔ per sq. in., the tensile working stress 50 ℔ per sq. in., and the working shearing stress 75 ℔ per sq. in. The tensile stress on the steel may be 16,000 ℔ per sq. in. The amount of steel in the structure may vary from 0.75 to 1.5%. The concrete not only affords much of the strength to resist compression, but effectively protects the steel from corrosion.
8. (c) Suspension Bridges.—A suspension bridge consists of two or more chains, constructed of links connected by pins, or of twisted wire strands, or of wires laid parallel. The chains pass over lofty piers on which they usually rest on saddles carried by rollers, and are led down on either side to anchorages in rock chambers. A level platform is hung from the chains by suspension rods. In the suspension bridge iron or steel can be used in its strongest form, namely hard-drawn wire. Iron suspension bridges began to be used at the end of the 18th century for road bridges with spans unattainable at that time in any other system. In 1819 T. Telford began the construction of the Menai bridge (fig. 10), the span being 570 ft. and the dip 43 ft. This bridge suffered some injury in a storm, but it is still in good condition and one of the most graceful of bridges. Other bridges built soon after were the Fribourg bridge of 870 ft. span, the Hammersmith bridge of 422 ft. span, and the Pest bridge of 666 ft. span. The merit of the simple suspension bridge is its cheapness, and its defect is its flexibility. This last becomes less serious as the dead weight of the structure becomes large in proportion to the live or temporary load. It is, therefore, a type specially suited for great spans. Some suspension bridges have broken down in consequence of the oscillations produced by bodies of men marching in step. In 1850 a suspension bridge at Angers gave way when 487 soldiers were marching over it, and 226 were killed.
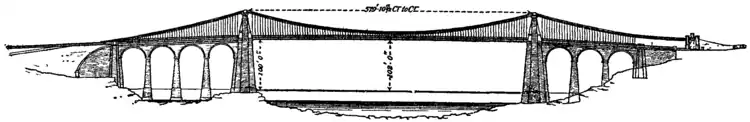
Fig. 10.—Menai Suspension Bridge.
To obtain greater stiffness various plans have been adopted. In the Ordish system a certain number of intermediate points in the span are supported by oblique chains, on which girders rest. The Ordish bridge built at Prague in 1868 had oblique chains supporting the stiffening girders at intermediate points of the span. A curved chain supported the oblique chains and kept them straight. In 1860 a bridge was erected over the Danube canal at Vienna, of 264 ft. span which had two parallel chains one above the other and 4 ft. apart on each side of the bridge. The chains of each pair were connected by bracing so that they formed a stiff inverted arch resisting deformation under unequal loading. The bridge carried a railway, but it proved weak owing to errors of calculation, and it was taken down in 1884. The principle was sound and has been proposed at various times. About 1850 it was perceived that a bridge stiff enough to carry railway trains could be constructed by combining supporting chains with stiffening girders suspended from them. W. J. M. Rankine proved (Applied Mechanics, p. 370) that the necessary strength of a stiffening girder would be only one-seventh part of that of an independent girder of the same span as the bridge, suited to carry the same moving load (not including the dead weight of the girder which is supported by the chain). (See “Suspension Bridge with Stiffened Roadway,” by Sir G. Airy, and the discussion, Proc. Inst. C.E., 1867, xxvi. p. 258; also “Suspension Bridges with Stiffening Girders,” by Max am Ende, Proc. Inst. C.E. cxxxvii. p. 306.)
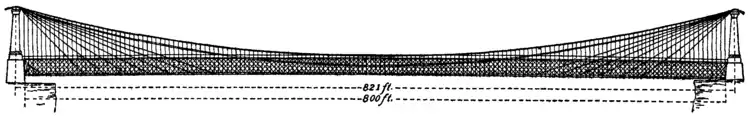
Fig. 11.—Niagara Suspension Bridge.
The most remarkable bridge constructed on this system was the Niagara bridge built by J. A. Roebling in 1852–1855 (fig. 11). The span was 821 ft., much the largest of any railway bridge at that time, and the height above the river 245 ft. There were four suspension cables, each 10 in. in diameter; each was composed of seven strands, containing 520 parallel wires, or 3640 wires in each cable. Each cable was carried on a separate saddle on rollers on each pier. The stiffening girder, constructed chiefly of timber, was a box-shaped braced girder 18 ft. deep and 25 ft. wide, carrying the railway on top and a roadway within. After various repairs and strengthenings, including the replacement of the timber girder by an iron one in 1880, this bridge in 1896–1897 was taken down and a steel arch built in its place. It was not strong enough to deal with the increasing weight of railway traffic. In 1836 I. K. Brunei constructed the towers and abutments for a suspension bridge of 702 ft. span at Clifton over the Avon, but the project was not then carried further; in 1860, however, the link chains of the Hungerford suspension bridge which was being taken down were available at small cost, and these were used to complete the bridge. There are three chains on each side, of one and two links alternately, and these support wrought iron stiffening girders. There are wrought iron saddles and steel rollers on the piers. At 196 ft. on either side from the towers the chains are carried over similar saddles without rollers, and thence at 45° with the horizontal down to the anchorages. Each chain has an anchor plate 5 ft. by 6 ft. The links are 24 ft. long at the centre of the bridge, and longer as they are more inclined, so that their horizontal projection is 24 ft. The chains are so arranged that there is a suspending rod at each 8 ft., attached at the joint of one of the three chains. For erection a suspended platform was constructed on eight wire ropes, on which the chains were laid out and connected. Another wire rope with a travelling carriage took out the links. The sectional area of the chains is 481 sq. in. at the piers and 440 sq. in. at the centre. The two stiffening girders are plate girders 3 ft. deep with flanges of 11 sq. in. area. In addition, the hand railing on each side forms a girder 4 ft. 9 in. deep, with flanges 412 sq. in. area.
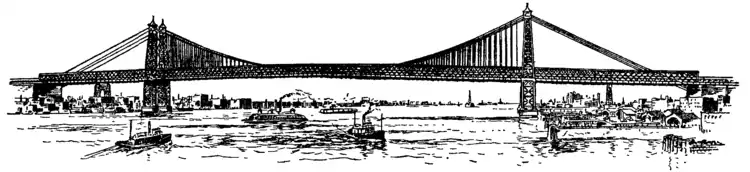
Fig. 12.—Williamsburg Suspension Bridge.
Of later bridges of great span, perhaps the bridges over the East river at New York are the most remarkable. The Brooklyn bridge, begun in 1872, has a centre span of 159512 and side spans of 930 ft. The Brooklyn approach being 971 ft., and the New York approach 156212 ft., the total length of the bridge is 5989 ft. There are four cables which carry a promenade, a roadway and an electric railway. The stiffening girders of the main span are 40 ft. deep and 67 ft. apart. The saddles for the chains are 329 ft. above high water. The cables are 1534 in. in diameter. Each cable has 19 strands of 278 parallel steel wires, 7 B.W.G. Each wire is taken separately across the river and its length adjusted. Roebling preferred parallel wires as 10% stronger than twisted wires. Each strand when made up and clamped was lowered to its position. The Williamsburg bridge (fig. 12), begun in 1897 and opened for traffic in 1903, has a span of 1600 ft., a versed sine of 176 ft., and a width of 118 ft. It has two decks, and carries two elevated railway tracks, four electric tramcar lines, two carriageways, two footways and two bicycle paths. There are four cables, one on each side of the two main trusses or stiffening girders. These girders are supported by the cables over the centre span but not in the side spans. Intermediate piers support the trusses in the side spans. The cables are 1834 in. in diameter; each weighs about 1116 tons, and has a nominal breaking strength of 22,320 tons, the actual breaking strength being probably greater. The saddles are 332 ft. above the water. The four cables support a dead load of 7140 tons and a live load of 4017 tons. Each cable is composed of 37 strands of 208 wires, or 7696 parallel steel wires, No. 8 B.W.G., or about 316 in. in diameter. The wire was required to have a tensile strength of 89 tons per sq. in., and 212% elongation in 5 ft. and 5% in 8 in. Cast steel clamps hold the cable together, and to these the suspending rods are attached. The cables are wrapped in cotton duck soaked in oxidized oil and varnish, and are sheathed in sheet iron. A later bridge, the Manhattan, is designed to carry four railway tracks and four tramway lines, with a wide roadway and footpaths, supported by cables 2114 in. in diameter, each composed of 9472 galvanized steel wires 316 in. in diameter.
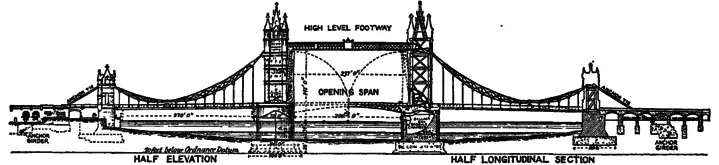
Fig. 13.—Tower Bridge, London.
The Tower Bridge, London (fig. 13), is a suspension bridge with a secondary bascule bridge in the centre span to permit the passage of ships. Two main towers in the river and two towers on the shore abutments carry the suspension chains. The opening bridge between the river towers consists of two leaves or bascules, pivoted near the faces of the piers and rotating in a vertical plane. When raised, the width of 200 ft. between the main river piers is unobstructed up to the high-level foot-bridge, which is 141 ft. above Trinity H.W. The clear width of the two shore spans is 270 ft. The total length of the bridge is 940 ft., and that of the approaches 1260 ft. on the north and 780 ft. on the south. The width of the bridge between parapets is 60 ft., except across the centre span, where it is 49 ft. The main towers consist of a skeleton of steel, enclosed in a facing of granite and Portland stone, backed with brickwork. There are two high-level footways for use when the bascules are raised, the main girders of which are of the cantilever and suspended girder type. The cantilevers are fixed to the shore side of the towers. The middle girders are 120 ft. in length and attached to the cantilevers by links. The main suspension chains are carried across the centre span in the form of horizontal ties resting on the high-level footway girders. These ties are jointed to the hanging chains by pins 20 in. in diameter with a ring in halves surrounding it 5 in. thick. One half ring is rigidly attached to the tie and one to the hanging chain, so that the wear due to any movement is distributed over the length of the pin. A rocker bearing under these pins transmits the load at the joint to the steel columns of the towers. The abutment towers are similar to the river towers. On the abutment towers the chains are connected by horizontal links, carried on rockers, to anchor ties. The suspension chains are constructed in the form of braced girders, so that they are stiff against unsymmetrical loading. Each chain over a shore span consists of two segments, the longer attached to the tie at the top of the river tower, the shorter to the link at the top of the abutment tower, and the two jointed together at the lowest point. Transverse girders are hung from the chains at distances of 18 ft. There are fifteen main transverse girders to each shore span, with nine longitudinal girders between each pair. The trough flooring, 38 in. thick and 6 in. deep, is riveted to the longitudinals. The anchor ties are connected to girders embedded in large concrete blocks in the foundations of the approach viaducts.
The two bascules are each constructed with four main girders. Over the river these are lattice girders, with transverse girders 12 ft. apart, and longitudinal and subsidiary transverse girders dividing the floor into rectangles 3 ft. by 312 ft. covered with buckled plates. The roadway is of pine blocks dowelled. The bascules rotate through an angle of 82°, and their rear ends in the bascule chambers of the piers carry 365 tons of counterweight, the total weight of each being 1070 tons. They rotate on steel shafts 21 in. in diameter and 48 ft. long, and the bascules can be lifted or lowered in one minute, but usually the time taken is one and a half minutes. They are worked by hydraulic machinery.
- ↑ For the ancient bridges in Rome see further Rome: Archaeology, and such works as R. Lanciani, Ruins and Excavations of Ancient Rome (Eng. trans., 1897), pp. 16 foll.