The Foucault pendulum or Foucault's pendulum is a simple device named after French physicist Léon Foucault, conceived as an experiment to demonstrate the Earth's rotation. A long and heavy pendulum suspended from the high roof above a circular area was monitored over an extended time period, showing that its plane of oscillation rotated.
The pendulum was introduced in 1851 and was the first experiment to give simple, direct evidence of the Earth's rotation. Foucault followed up in 1852 with a gyroscope experiment to further demonstrate the Earth's rotation. Foucault pendulums today are popular displays in science museums and universities.[1]
Original Foucault pendulum

Foucault was inspired by observing a thin flexible rod on the axis of a lathe, which vibrated in the same plane despite the rotation of the supporting frame of the lathe.[2]
The first public exhibition of a Foucault pendulum took place in February 1851 in the Meridian of the Paris Observatory. A few weeks later, Foucault made his most famous pendulum when he suspended a 28-kilogram (62 lb) brass-coated lead bob with a 67-metre long (220 ft) wire from the dome of the Panthéon, Paris.
Because the latitude of its location was , the plane of the pendulum's swing made a full circle in approximately , rotating clockwise approximately 11.3° per hour. The proper period of the pendulum was approximately , so with each oscillation, the pendulum rotates by about . Foucault reported observing 2.3 mm of deflection on the edge of a pendulum every oscillation, which is achieved if the pendulum swing angle is 2.1°.[2]
Foucault explained his results in an 1851 paper entitled Physical demonstration of the Earth's rotational movement by means of the pendulum, published in the Comptes rendus de l'Académie des Sciences. He wrote that, at the North Pole:[3]
...an oscillatory movement of the pendulum mass follows an arc of a circle whose plane is well known, and to which the inertia of matter ensures an unchanging position in space. If these oscillations continue for a certain time, the movement of the earth, which continues to rotate from west to east, will become sensitive in contrast to the immobility of the oscillation plane whose trace on the ground will seem animated by a movement consistent with the apparent movement of the celestial sphere; and if the oscillations could be perpetuated for twenty-four hours, the trace of their plane would then execute an entire revolution around the vertical projection of the point of suspension.
The original bob used in 1851 at the Panthéon was moved in 1855 to the Conservatoire des Arts et Métiers in Paris. A second temporary installation was made for the 50th anniversary in 1902.[4]
During museum reconstruction in the 1990s, the original pendulum was temporarily displayed at the Panthéon (1995), but was later returned to the Musée des Arts et Métiers before it reopened in 2000.[5] On April 6, 2010, the cable suspending the bob in the Musée des Arts et Métiers snapped, causing irreparable damage to the pendulum bob and to the marble flooring of the museum.[6][7] The original, now damaged pendulum bob is displayed in a separate case adjacent to the current pendulum display.
An exact copy of the original pendulum has been operating under the dome of the Panthéon, Paris since 1995.[8]
Explanation of mechanics
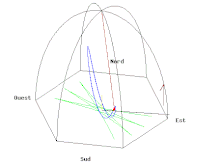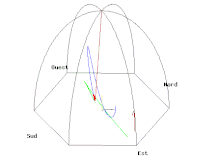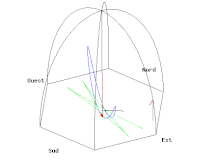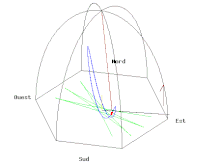
At either the Geographic North Pole or Geographic South Pole, the plane of oscillation of a pendulum remains fixed relative to the distant masses of the universe while Earth rotates underneath it, taking one sidereal day to complete a rotation. So, relative to Earth, the plane of oscillation of a pendulum at the North Pole – viewed from above – undergoes a full clockwise rotation during one day; a pendulum at the South Pole rotates counterclockwise.
When a Foucault pendulum is suspended at the equator, the plane of oscillation remains fixed relative to Earth. At other latitudes, the plane of oscillation precesses relative to Earth, but more slowly than at the pole; the angular speed, ω (measured in clockwise degrees per sidereal day), is proportional to the sine of the latitude, φ:
where latitudes north and south of the equator are defined as positive and negative, respectively. A "pendulum day" is the time needed for the plane of a freely suspended Foucault pendulum to complete an apparent rotation about the local vertical. This is one sidereal day divided by the sine of the latitude.[10][11] For example, a Foucault pendulum at 30° south latitude, viewed from above by an earthbound observer, rotates counterclockwise 360° in two days.

Using enough wire length, the described circle can be wide enough that the tangential displacement along the measuring circle of between two oscillations can be visible by eye, rendering the Foucault pendulum a spectacular experiment: for example, the original Foucault pendulum in Panthéon moves circularly, with a 6-metre pendulum amplitude, by about 5 mm each period.

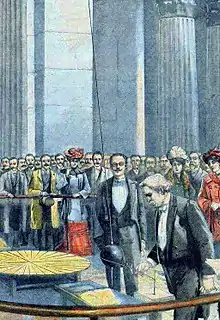
A Foucault pendulum requires care to set up because imprecise construction can cause additional veering which masks the terrestrial effect. Heike Kamerlingh Onnes (Nobel laureate 1913) performed precise experiments and developed a fuller theory of the Foucault pendulum for his doctoral thesis (1879). He observed the pendulum to go over from linear to elliptic oscillation in an hour. By a perturbation analysis, he showed that geometrical imperfection of the system or elasticity of the support wire may cause a beat between two horizontal modes of oscillation.[12] The initial launch of the pendulum is also critical; the traditional way to do this is to use a flame to burn through a thread which temporarily holds the bob in its starting position, thus avoiding unwanted sideways motion (see a detail of the launch at the 50th anniversary in 1902).
Notably, veering of a pendulum was observed already in 1661 by Vincenzo Viviani, a disciple of Galileo, but there is no evidence that he connected the effect with the Earth's rotation; rather, he regarded it as a nuisance in his study that should be overcome with suspending the bob on two ropes instead of one.
Air resistance damps the oscillation, so some Foucault pendulums in museums incorporate an electromagnetic or other drive to keep the bob swinging; others are restarted regularly, sometimes with a launching ceremony as an added attraction. Besides air resistance (the use of a heavy symmetrical bob is to reduce friction forces, mainly air resistance by a symmetrical and aerodynamic bob) the other main engineering problem in creating a 1-meter Foucault pendulum nowadays is said to be ensuring there is no preferred direction of swing.[13]
Related physical systems

Many physical systems precess in a similar manner to a Foucault pendulum. As early as 1836, the Scottish mathematician Edward Sang contrived and explained the precession of a spinning top. In 1851, Charles Wheatstone[14] described an apparatus that consists of a vibrating spring that is mounted on top of a disk so that it makes a fixed angle φ with the disk. The spring is struck so that it oscillates in a plane. When the disk is turned, the plane of oscillation changes just like the one of a Foucault pendulum at latitude φ.
Similarly, consider a nonspinning, perfectly balanced bicycle wheel mounted on a disk so that its axis of rotation makes an angle φ with the disk. When the disk undergoes a full clockwise revolution, the bicycle wheel will not return to its original position, but will have undergone a net rotation of 2π sin φ.
Foucault-like precession is observed in a virtual system wherein a massless particle is constrained to remain on a rotating plane that is inclined with respect to the axis of rotation.[15]
Spin of a relativistic particle moving in a circular orbit precesses similar to the swing plane of Foucault pendulum. The relativistic velocity space in Minkowski spacetime can be treated as a sphere S3 in 4-dimensional Euclidean space with imaginary radius and imaginary timelike coordinate. Parallel transport of polarization vectors along such sphere gives rise to Thomas precession, which is analogous to the rotation of the swing plane of Foucault pendulum due to parallel transport along a sphere S2 in 3-dimensional Euclidean space.[16]
In physics, the evolution of such systems is determined by geometric phases.[17][18] Mathematically they are understood through parallel transport.
Foucault pendulums around the world
There are numerous Foucault pendulums at universities, science museums, and the like throughout the world. The United Nations General Assembly Building at the United Nations headquarters in New York City has one. The Oregon Convention Center pendulum is claimed to be the largest, its length approximately 27 m (89 ft),[19][20] however, there are larger ones listed in the article, such as the one in Gamow Tower at the University of Colorado (39.3 m). There used to be much longer pendulums, such as the 98 m (322 ft) pendulum in Saint Isaac's Cathedral, Saint Petersburg, Russia.[21][22]
 Foucault pendulum at the Musée des Arts et Métiers
Foucault pendulum at the Musée des Arts et Métiers Foucault pendulum at the Ranchi Science Centre
Foucault pendulum at the Ranchi Science Centre Foucault pendulum at the California Academy of Sciences
Foucault pendulum at the California Academy of Sciences Foucault pendulum at the Devonshire Dome, University of Derby
Foucault pendulum at the Devonshire Dome, University of Derby Foucault's Pendulum in the stairwell of Franklin Institute (Photo taken on May 28, 2012)
Foucault's Pendulum in the stairwell of Franklin Institute (Photo taken on May 28, 2012)
South Pole
The experiment has also been carried out at the South Pole, where it was assumed that the rotation of the Earth would have maximum effect.[23][24] A pendulum was installed in a six-story staircase of a new station under construction at the Amundsen-Scott South Pole Station. It had a length of 33 m (108 ft) and the bob weighed 25 kg (55 lb). The location was ideal: no moving air could disturb the pendulum. The researchers confirmed about 24 hours as the rotation period of the plane of oscillation.
See also
References
- ↑ Oprea, John (1995). "Geometry and the Foucault Pendulum". Amer. Math. Monthly. 102 (6): 515–522. doi:10.2307/2974765. JSTOR 2974765. Archived from the original on 2015-04-02.
- 1 2 Sommeria, Joël (2017-11-01). "Foucault and the rotation of the Earth". Comptes Rendus Physique. Science in the making: The Comptes rendus de l’Académie des sciences throughout history. 18 (9): 520–525. Bibcode:2017CRPhy..18..520S. doi:10.1016/j.crhy.2017.11.003. ISSN 1631-0705.
- ↑ Foucault, Léon (1851). (in French) – via Wikisource.
- ↑ "The Pendulum of Foucault of the Panthéon. Ceremony of inauguration by M. Chaumié, minister of the state education, burnt the wire of balancing, to start the pendulum. 1902". Paris en images. Archived from the original on 2014-08-21.
- ↑ Kissell, Joe (November 8, 2004). "Foucault's Pendulum: Low-tech proof of Earth's rotation". Interesting thing of the day. Archived from the original on March 12, 2012. Retrieved March 21, 2012.
- ↑ Thiolay, Boris (April 28, 2010). "Le pendule de Foucault perd la boule". L'Express (in French). Archived from the original on July 10, 2010.
- ↑ "Foucault's pendulum is sent crashing to Earth". Times Higher Education. 13 May 2010. Archived from the original on 20 March 2012. Retrieved March 21, 2012.
- ↑ "Foucault's Pendulum and the Paris Pantheon". Atlas Obscura. Archived from the original on January 12, 2018. Retrieved January 12, 2018.
- ↑ "Foucault Pendulum". Smithsonian Encyclopedia. Retrieved September 2, 2013.
- ↑ "Pendulum day". Glossary of Meteorology. American Meteorological Society. Archived from the original on 2007-08-17.
- ↑ Daliga, K.; Przyborski, M.; Szulwic, J. "Foucault's Pendulum. Uncomplicated Tool In The Study Of Geodesy And Cartography". library.iated.org. Archived from the original on 2016-03-02. Retrieved 2015-11-02.
- ↑ Sommeria, Joël (1 November 2017). "Foucault and the rotation of the Earth". Comptes Rendus Physique. 18 (9): 520–525. Bibcode:2017CRPhy..18..520S. doi:10.1016/j.crhy.2017.11.003.
- ↑ "A Short, Driven, Foucault Pendulum". Archived from the original on 2009-03-31.
- ↑ Charles Wheatstone Wikisource: "Note relating to M. Foucault's new mechanical proof of the Rotation of the Earth", pp. 65–68.
- ↑ Bharadhwaj, Praveen (2014). "Foucault precession manifested in a simple system". arXiv:1408.3047 [physics.pop-ph].
- ↑ Krivoruchenko, M. I. (2009). "Rotation of the swing plane of Foucault's pendulum and Thomas spin precession: Two faces of one coin". Phys. Usp. 52 (8): 821–829. arXiv:0805.1136. Bibcode:2009PhyU...52..821K. doi:10.3367/UFNe.0179.200908e.0873. S2CID 118449576.
- ↑ "Geometric Phases in Physics", eds. Frank Wilczek and Alfred Shapere (World Scientific, Singapore, 1989).
- ↑ L. Mangiarotti, G. Sardanashvily, Gauge Mechanics (World Scientific, Singapore, 1998)
- ↑ "Kristin Jones - Andrew Ginzel". Retrieved 5 May 2018.
- ↑ "LTW Automation Products". ltwautomation.net. Archived from the original on 29 April 2016. Retrieved 5 May 2018.
- ↑ "The first Foucault pendulum in Russia, beyond the Arctic Circle". 2018-06-14. Archived from the original on 2019-03-21. Retrieved 2019-03-21.
- ↑ Great Soviet Encyclopedia
- ↑ Johnson, George (September 24, 2002). "Here They Are, Science's 10 Most Beautiful Experiments". The New York Times. Archived from the original on May 31, 2012. Retrieved September 20, 2012.
- ↑ Baker, G. P. (2011). Seven Tales of the Pendulum. Oxford University Press. p. 388. ISBN 978-0-19-958951-7.
Further reading
- Arnold, V.I. (1989). Mathematical Methods of Classical Mechanics. Springer. p. 123. ISBN 978-0-387-96890-2.
- Marion, Jerry B.; Thornton, Stephen T. (1995). Classical dynamics of particles and systems (4th ed.). Brooks Cole. pp. 398–401. ISBN 978-0-03-097302-4.
- Persson, Anders O. (2005). "The Coriolis Effect: Four centuries of conflict between common sense and mathematics, Part I: A history to 1885" (PDF). History of Meteorology. 2. Archived from the original (PDF) on 2014-04-11. Retrieved 2006-04-27.
External links
- Wolfe, Joe, "A derivation of the precession of the Foucault pendulum".
- "The Foucault Pendulum", derivation of the precession in polar coordinates.
- "The Foucault Pendulum" By Joe Wolfe, with film clip and animations.
- "Foucault's Pendulum" by Jens-Peer Kuska with Jeff Bryant, Wolfram Demonstrations Project: a computer model of the pendulum allowing manipulation of pendulum frequency, Earth rotation frequency, latitude, and time.
- "Webcam Kirchhoff-Institut für Physik, Universität Heidelberg".
- California academy of sciences, CA Archived 2016-05-25 at the Portuguese Web Archive Foucault pendulum explanation, in friendly format
- Foucault pendulum model Exposition including a tabletop device that shows the Foucault effect in seconds.
- Foucault, M. L., Physical demonstration of the rotation of the Earth by means of the pendulum, Franklin Institute, 2000, retrieved 2007-10-31. Translation of his paper on Foucault pendulum.
- Tobin, William. "The Life and Science of Léon Foucault".
- Bowley, Roger (2010). "Foucault's Pendulum". Sixty Symbols. Brady Haran for University of Nottingham.
- Pendolo nel Salone The Foucault Pendulum inside Palazzo della Ragione in Padova, Italy
- Chessin, A. S. (1895). "On Foucault's Pendulum". Am. J. Math. 17 (1): 81–88. doi:10.2307/2369710. JSTOR 2369710.
- Bromwich, T. J. (1914). "The theory of Foucault's pendulum". Proc. Lond. Math. Soc. s2-13 (1): 222–235. doi:10.1112/plms/s-13.1.222.
- MacMillan, William Duncan (1915). "On Foucault's Pendulum". Am. J. Math. 37 (1): 95–106. doi:10.2307/2370259. JSTOR 2370259. S2CID 123717776.
- Noble, William J. (1952). "A direct treatment of the Foucault Pendulum". Am. J. Phys. 20: 334–336. doi:10.1119/1.1933230.
- Schulz-DuBois, E. O. (1970). "Foucault Pendulum Experiment by Kamerlingh Onnes and degenerate perturbation theory". Am. J. Phys. 38: 173. doi:10.1119/1.1976270.
- Somerville, W. B. (1972). "The description of Foucault's pendulum". Q. J. R. Astron. Soc. 13: 40–62. Bibcode:1972QJRAS..13...40S.
- Braginsky, Vladimir B.; Polnarev, Aleksander G.; Thorne, Kip S. (1984). "Foucault Pendulum at the South Pole: Proposal For an Experiment to Detect the Earth's General Relativistic Gravitomagnetic Field" (PDF). Phys. Rev. Lett. 53 (9): 863. Bibcode:1984PhRvL..53..863B. doi:10.1103/PhysRevLett.53.863.
- Hard, John B.; Miller, Raymond E. (1987). "A simple geometric model for visualizing the motion of a Foucault pendulum". Am. J. Phys. 55 (1): 67. Bibcode:1987AmJPh..55...67H. doi:10.1119/1.14972.
- Crane, H. Richard (1995). "Foucault pendulum "wall clock"". Am. J. Phys. 63 (1): 33–39. Bibcode:1995AmJPh..63...33C. doi:10.1119/1.17765.
- Das, U.; Talukdar, B.; Shamanna, J. (2002). "Indirect Analytic Representation of Foucault's Pendulum". Czechoslov. J. Phys. 52 (12): 1321–1327. Bibcode:2002CzJPh..52.1321D. doi:10.1023/A:1021819627736. S2CID 118592240.
- Salva, Horacio R.; Benavides, Rubén E.; Perez, Julio C.; Cuscueta, Diego J. (2010). "A Foucault's pendulum design". Rev. Sci. Instrum. 81 (11): 115102–115102–4. Bibcode:2010RScI...81k5102S. doi:10.1063/1.3494611. PMID 21133496.
- de Icaza-Herrera, M.; Castano, V. M. (2011). "Generalized Lagrangian of the parametric Foucault pendulum with dissipative forces". Acta Mech. 218: 45–64. doi:10.1007/s00707-010-0392-8.
- Daliga, K.; Przyborski, M.; Szulwic, J. (2015). "Foucault's Pendulum. Uncomplicated Tool in the Study of Geodesy and Cartography". EDULEARN15 Proceedings - 7th International Conference on Education and New Learning Technologies, Barcelona, Spain. IATED Academy. ISBN 978-84-606-8243-1.
- Cartmell, Matthew P.; Faller, James E.; Lockerbie, Nicholas A.; Handous, Eva (2020). "On the modelling and testing of a laboratory-scale Foucault pendulum as a precursor for the design of a high-performance measurement instrument". Proc. Roy. Soc. A. 476 (2238): 20190680. doi:10.1098/rspa.2019.0680. PMC 7428043.
